When a Knot Is Not a Knot
Experiment
Even mathematicians—studying the rules that underlie reality—are tied up in knots over the surprising twists of “knot theory.”
A stressed-out person may be described as “tied in knots,” unable to relax or make good decisions. Mathematicians, in contrast, find knots fascinating, and some of them devote their lives to the math of knots, including computer modeling to understand all their twists and turns. It may sound like an armchair interest with no practical value, but read on.
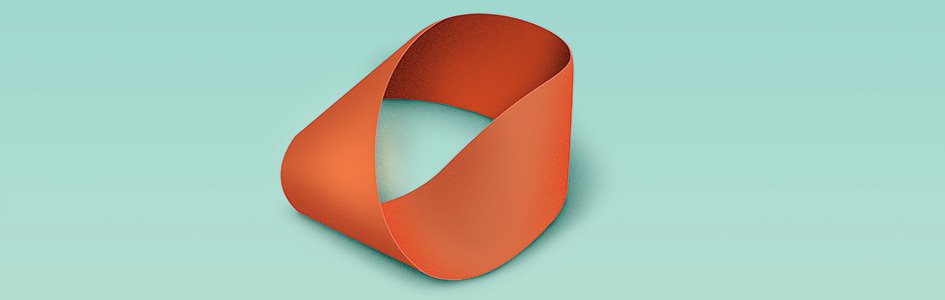
One of the great pioneer knot explorers was August Ferdinand Möbius (1790–1868), a descendent of Martin Luther. Homeschooled and gifted in mathematics, Möbius is best known for his study of a twisted belt of material called a Möbius strip. The unusual figure somewhat resembles a lazy eight or infinity symbol.
The Möbius shape has several fascinating properties, and the reader is encouraged to experiment with a ribbon of paper to see it for himself. When constructed, the two surfaces of the paper ribbon become joined to form a ring with just a single side, strange but true.
By twisting and tearing a Möbius strip in various ways, you can create several new novel forms of ribbon. The technical details of Möbius ribbons are analyzed in the field of mathematics called topology, which has significant uses in modern industry, computers, and even particle physics.
The Möbius shape has had several practical applications since its discovery. For example, a large Möbius band makes a good conveyor belt. It will last twice as long as a conventional belt because it wears evenly along its entire length, both topside and underside. Farm machinery also may use the Möbius concept of a twisted belt for longer wear.
Some continuous-loop audiotapes employ the Möbius shape to double their recording time. The Möbius shape is also popular in sculpture and graphic art, especially the work of M. C. Escher (1898–1972), who was intrigued by its nearly magical, otherworldly effects that seemed to defy basic geometry.
Though Möbius is credited with discovering this unusual shape, it already occurs in nature. For example, some of the vast circulating ocean currents follow the path of a Möbius loop. The metallic compound niobium selenide, NbSe3, assumes the twisted curve shape when grown in its crystalline form. Certain organic molecules and segments of DNA likewise can adopt a Möbius pattern or other types of “molecular knots.” These result in materials with new physical properties and possible applications in miniaturized electronic devices.
Mathematicians define knots differently from the knots we see in everyday life, such as shoelaces or sailing knots. A mathematical knot has no loose ends to tie up; instead, it is made up of a closed loop that may be entangled in various ways. If the loop can be untangled into an open circle, it is known as an “unknot.” (Unknots have their own incredible properties.)
Several creatures are adept at knots:
The moray eel can tie itself into a knot when it needs to hold its body in place on the seafloor or in a cave.
The hagfish, similar to the eel, can tie itself into a knot, which slides down the body, cleaning the skin surface.
Weaver birds tie knots in nest material using their beaks and feet.
In contrast to these knot-makers, octopuses have special instincts to avoid knotting their eight limbs.
Analysis of the Möbius shape and related animal behavior reveals the deep mathematics that underlies all of creation. This elegant and complex language was established during the Creation Week.
Job 12:7–9 challenges us to speak to the earth, animals, birds, and fish, “and they will teach you.” That is, God expects us to survey and study His world, including the birds, fish, and other animals, to learn more about our Creator and how He operates. All of creation declares the hand of the Lord, including the knots we find in nature.
Answers Magazine
April–June 2016
How well do you know the views of modern creationists? Test your knowledge and then discover how Christians can effectively share the gospel with homosexuals.
Browse Issue SubscribeRecommended Resources

Answers in Genesis is an apologetics ministry, dedicated to helping Christians defend their faith and proclaim the good news of Jesus Christ.
- Customer Service 800.778.3390
- © 2025 Answers in Genesis