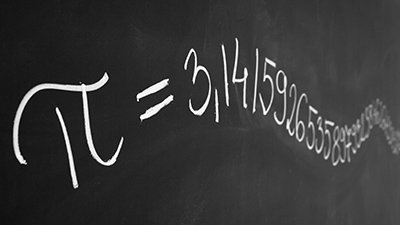Celebrate Einstein’s Birthday with Pi on 3.14.15

Today is March 14, 2015 or 3.14.15. That string of numbers looks familiar to many of us because they are the first five digits of pi: 3.1415. Pi (π) is a constant and has a list of digits that goes on for what may be infinity. It’s the ratio of the circumference of a circle to its diameter. Having the dates of the calendar match with the numbers of pi including the abbreviated year only happens once a century on March 14.

This date is also unique because it’s the famous physicist Dr. Albert Einstein’s birthday today (March 14, 1879–April 18, 1955). Einstein is remembered most for his general theory of relativity, or his mass-energy equivalence (E=mc²), which has helped scientists to better understand and predict how the universe works.

A True and Consistent Basis

Physics and mathematics are only possible because we live in a rational universe. If random naturalistic evolution were true, then we shouldn’t expect to have universal constants like pi. Why should things work the same throughout the whole universe? Why should our universe run in an orderly fashion if it is just the result of purposeless chance? What gives order to our whole universe? What causes pi to be the same today and tomorrow? Why do the laws of physics operate in predictable ways?

We live in an orderly and consistent universe because there is a consistent God who upholds the universe (Hebrews 1:3). Universal constants and order make sense because there is a God who never changes (Malachi 3:6) and who has imposed order on His creation—and this all-knowing God has informed us of this. That’s why we can know that the laws of nature will operate the same way next week as they did this week (Genesis 8:22).

In order for us to even be able to do physics or mathematics, we must assume that the universe is orderly and that laws of nature will operate the same tomorrow as today. Yet in a naturalistic worldview there is no way to know the future . . . for all they know, the laws of nature might change tomorrow.

Math and Autonomy

As “concrete” as math seems to be, the works of Kurt Gödel (1906–1978), who incidentally was a friend of Einstein, and Luitzen Brouwer (1882–1966) have affected this past assumption. Gödel’s two Incompleteness Theorems basically state that no logical systems that include counting numbers can have all three of the following properties:

1. Validity . . . all conclusions are reached by valid reasoning.
2. Consistency . . . no conclusions contradict any other conclusions.
3. Completeness . . . all statements made in the system are either true or false.1

In effect, logic was used to prove that mathematics could not be autonomous, opening the door to a Creator outside “the system” that could instead be the basis of autonomy. Obviously, laws of logic are required to exist to make math possible. And a logical God must exist to make laws of logic possible as even the universe obeys laws of logic (i.e., you can’t have the moon and not the moon at the same time and the same relationship).

Later Brouwer “rejected the idea that math is discovered, and he promoted instead the view that math is invented by men. In his view, the human mind is the foundation of math instead of God.”2 This philosophy, called Intuitionism, is favored by many mathematicians, but if math is an invention of many human minds, then why should there be any consistency and how could it be so ubiquitous and useful? But this just pushed the conceptual problem of mathematics to another source anyway. On what basis then does the conceptual human mind exist in a materialistic worldview?

The fact that there are still areas of math that are a mystery, such as the Incompleteness Theorem, the supposed problem with the order of prime numbers, and that irrational numbers and infinity cannot be truly comprehended, is not a problem at all when we look to a boundless Creator who not only stands outside such conventions, but indeed created them! Logic, physics, and math are for our use—and use them we do without fear of incompatibility with others using those same literally God-given tools, whether they believe in the source of those tools or not! And whether or not the observer chooses to acknowledge it, God’s character, which He’s revealed in part to our finite minds in Scripture, is the basis for both exploring and understanding His creation (Psalm 8). And despite mysterious aspects about prime numbers and π, there are eloquent associations with both of them that point to some order beyond our comprehension at this point.3

Objections

Some say the Bible has an error in relation to π. First Kings 7:23 states, “Now he made the sea of cast metal ten cubits from brim to brim, circular in form, and its height was five cubits, and thirty cubits in circumference.” If we divide the circumference (30 cubits) by the diameter (10 cubits), we come up with 3, not 3.1415 . . . , so is the Bible writer wrong or ignorant of the true value of π? Given that all Scripture is “God-breathed” (2 Timothy 3:16), this cannot be the case, so we must look elsewhere for the answer, which is surprisingly simple. The Bible writer here merely used rounding. Most people, if asked what the circumference of a cylinder with a diameter of 10 feet is (regardless of the thickness of the wall), would answer “about 30 feet.” Critics often grasp at straws to find Bible inaccuracies that simply aren’t there.4

Critics also accuse creation scientists of being anti-science for rejecting evolution and claim that America will fall behind in technology and innovation if we raise children to believe in a Creator of the Bible and in the history recorded in His Word. This misunderstanding really comes from a misunderstanding of the nature of science. There are two different kinds of science: observational and historical science. Observational science deals with the present and is directly testable, observable, and repeatable. Mathematics falls into this category. Any scientist can repeat the calculations of another scientist, and, assuming they do it correctly, they will get the same answer. Historical science, however, deals with the past and is therefore not directly testable, repeatable, or observable. What you believe about the past determines how you interpret the observational evidence. Now mathematical principles may be used when we are looking at and trying to interpret historical science, because math is a tool with principles that were true in the past and are true in the present. So while creationists and evolutionists agree on how to do the math and we both use exactly the same principles, we will not agree on how to interprt the past because we have different starting points.

God’s Handiwork and Character Revealed

Mathematics, like all branches of science, confirms God’s character and God Word. Indeed, no matter where we look in the universe or what branch of science we use, God’s Word is confirmed over and over again because it is true from the very beginning (Psalm 119:160). God is the Creator who has carefully fashioned this universe, imposed order on it, and upholds it (Colossians 1:16–17). Pi, logic, physics, math, and “the heavens declare the glory of God” (Psalm 19:1)—no amount of math, science, or technology has ever falsified that. We can trust His Word from the very beginning.