Flat Earth-Star Trails

In late May, an article that I wrote about the flat earth theory appeared on the Answers in Genesis website. I wondered what kind of response that it would provoke. Rob Skiba, one of the people that I mentioned, recently took note of the article. Skiba stated that what I wrote was a blog. Technically, it wasn’t. What I’m writing here is a blog, but that is a minor point. Skiba noted that the title of my article was “Is the Earth Flat?” and that my “answer to that question was essentially, ‘No. The Earth is not flat and the Bible never said it was!’” Skiba must not have read my article very carefully, because that is a bad characterization of what I wrote. For instance, I gave several reasons for why we know that the earth is spherical:

• The earth’s shadow during a lunar eclipse is round
• The stars visible in the sky change as one changes latitude
• Lunar eclipses happen at different times at different longitudes
• Distant objects over water are not visible at water level but are visible with some elevation

I selected these four evidences for a spherical earth because these are not modern arguments. People more than two thousand years ago used these arguments. Furthermore, people today easily can repeat these sorts of observations for themselves. This point is important because it is completely contrary to how Skiba went on to characterize my article:

Nor did they [speaking of Answers in Genesis] do much more than prove just how much they actually trust modern science (falsely so-called as Paul would say) and the Nazi/Freemason and thoroughly occult/pagan founded organization called NASA. The majority of their arguments stem from the preconceived notion that NASA is completely trustworthy, thus “we know that . . . blah, blah, blah . . . .”

In my article I never invoked the name of NASA. That term appears five times in the text of my article, and all five times it was in the context of discussing another flat earth proponent, Matthew Boylan, who claims to have done work for NASA. If I had not mentioned Boylan, the term NASA would not have even appeared in the article (though NASA is credited with two photos that illustrate the article). I even downplayed appealing to photographs from space showing that the earth is round, because such photos could be faked, a point that Skiba and other flat earth proponents consistently make.

Besides falsely accusing me of relying upon NASA, Skiba totally ignored the positive arguments for a spherical earth that I discussed. Furthermore, he failed to acknowledge that I rebutted several of the supposed evidences that supporters of the flat earth theory often put forward:

• Rowbotham’s 1838 Bedford Level experiment
• False claim that if the earth were spherical the only place that the midnight sun would be visible was the North Pole
• False claim that parallax has not been observed
• False claim that the North Star is visible down to 23 ½ degrees south latitude
• False claim that we can see stars through the moon
• False claim that rockets can’t work in space

Skiba mockingly criticized my mention of Christian astronauts Jeff Williams, Jim Irwin, and Charlie Duke:

Oh yeah, and Jim Irwin was a Christian (and also a Freemason btw), so how dare we question the words of other Christians!?

Part of the flat earth theory is that there are no satellites orbiting the earth, we have not sent people into space, and we have not landed men on the moon. With respect to Christian astronauts, the question that I asked of those who believe that the earth is flat is this:

To doubt that the earth is spherical or that astronauts have gone into space is to accuse a Christian brother of perpetuating a tremendous lie. . . . Christians who think that the earth is flat or that men never set foot on the moon are effectively accusing several Christian brothers of lying about one of the biggest things that ever happened in their lives. Are the Apollo moon landing deniers prepared to make this accusation?

From his brash response, Skiba apparently is prepared to make this accusation. As a professional astronomer, I certainly would be in a position to know about the earth’s true shape too. Therefore, Skiba ought to include me as part of his claims of a vast conspiracy of deceit. At any rate, in what follows, I draw upon my expertise in astronomy to explore some important factors that have bearing on the question of the earth’s shape.

The Appearance of the Nighttime Sky

The nighttime sky has the appearance of an inverted bowl. This is why planetariums have hemispherical ceilings—a planetarium projector at the hemisphere’s center gives a good representation of what the night sky looks like. As the earth spins, stars rise and set, so it is easy to see that the visible hemisphere is just half of a sphere, with the other hemisphere below the horizon. We can imagine that the stars are affixed to a sphere with a large radius surrounding the earth. Astronomers call this the celestial sphere. Of course, stars are not on a sphere because stars are not the same distance away from us. While the celestial sphere is an imaginary concept, we find it useful to describe certain aspects of the sky.

For instance, Figure 1 shows the celestial sphere surrounding the earth. Note that the sizes of the earth and the celestial sphere are not to scale. The distance to the closest star (other than the sun) is six billion times the earth’s radius, so the radius of the celestial sphere is at least six billion times the earth’s radius. Obviously, if the figure were to scale, the earth would not show up at all. Due to the earth’s rotation, the celestial sphere appears to spin around the earth each day. Just as the earth spins on an axis passing through the earth’s North Pole and South Pole, the celestial sphere appears to rotate each day around an axis passing through its north celestial pole and its south celestial pole. The celestial poles are the points where the earth’s axis intersects the celestial sphere. Halfway between the celestial poles is the celestial equator. The celestial equator is the intersection of the earth’s equator with the celestial sphere.

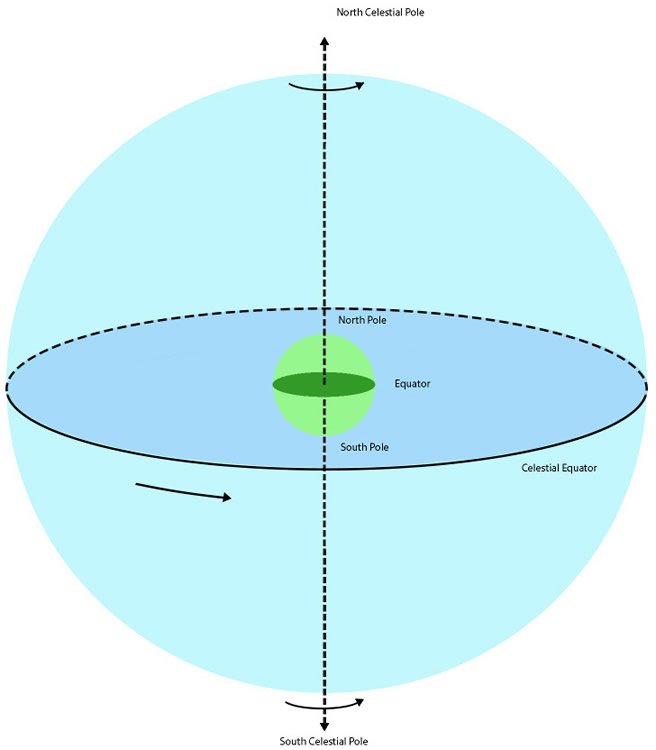
Figure 1.

To an observer at the North Pole (on top of the earth in Figure 1), the north celestial pole is at the zenith, the point directly overhead. The celestial equator lies along the horizon. Everything south of the celestial equator is below the horizon, because the earth would block the view of anything below the celestial equator. As the earth rotates, the sky appears to spin around the north celestial pole. Therefore, all stars that are above the celestial equator are always above the horizon, while stars below the celestial equator are always below the horizon. Stars that never rise or set are said to be circumpolar, meaning around the pole. From any location, there generally are two circumpolar regions, one region whose stars are always visible, and another whose stars are never visible (the exception is the earth’s equator – from locations on the equator, there are no circumpolar regions). At the North Pole, stars above the celestial equator are always visible, placing them in the visible circumpolar region. Stars below the celestial equator are in the non-visible circumpolar region. The situation is reversed at the South Pole: the south celestial pole is at the zenith. Stars below the celestial equator are always visible, and stars above the celestial equator never are visible.

Figure 2 illustrates the situation for an observer located in the Northern Hemisphere at latitude φ. The north celestial pole makes an angle φ with the northern horizon. As the celestial sphere spins, stars within angle φ of the north celestial pole are always above the horizon. This defines the visible circumpolar region. Likewise, stars within angle φ of the south celestial pole are never above the horizon, defining the non-visible circumpolar region. The celestial equator intersects the horizon due east and west, making an angle equal to the complement of the latitude. Stars not in either circumpolar region rise at some angle along the eastern horizon and set at the same angle along the western horizon. A similar thing happens in the Southern Hemisphere, but the south celestial pole appears above the south direction, making an angle equal to one’s latitude with the visible circumpolar region centered on the south celestial pole.

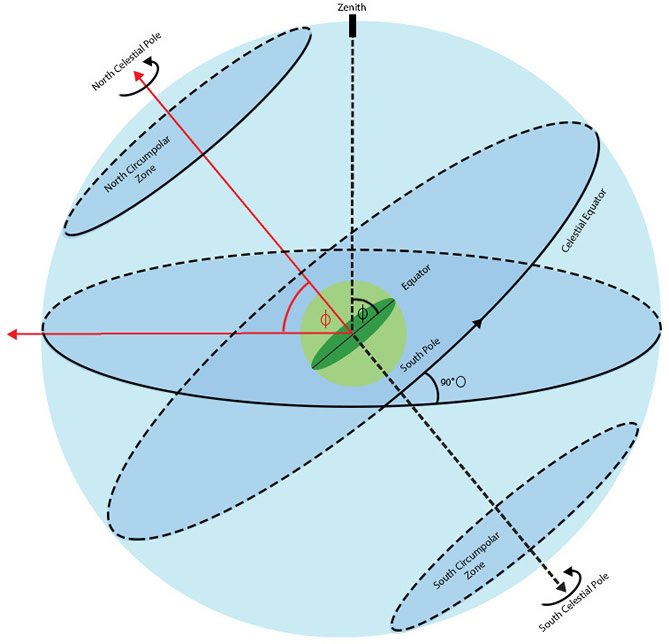
Figure 2.

As one moves northward, the latitude increases so that the angle that the north celestial pole makes with the northern horizon increases. There is a corresponding loss of stars visible to the south. Thus, both circumpolar regions increase in size. This change in appearance occurs because the earth is spherical. People in the ancient world were familiar with this phenomenon, and this was one of the arguments that ancient people, such as Aristotle, gave for the earth’s spherical shape.

Contrast this to the flat earth model, as illustrated in figure 3. In the flat earth model, the earth is round and flat with the North Pole at its center. There is no South Pole. Above the earth there is a dome on which the stars and other astronomical bodies are located. The north celestial pole is directly above the earth’s North Pole. The dome of the sky is thousands of miles in radius, comparable in size to the radius of the flat earth.1 The earth does not rotate, but rather the dome of the sky spins around the north celestial pole each day. Because the dome of the sky is only thousands of miles across, an observer, such as at point O, will see the north celestial pole at an angle φ above the northern horizon. At the North Pole φ will be a right angle, but φ will decrease with increasing distance from the North Pole. Notice that φ will always be greater than zero degrees, so the north celestial pole will be visible from all locations on the earth. Notice that since a line may be drawn from any point on the flat earth to any point on the dome of the sky, the entire dome is visible from all locations on the earth. That is, there are no stars that are visible from some locations but not from others. However, at different locations, particular stars will be visible in different parts of the sky. There is no south celestial pole, so all motion will be around the north celestial pole.

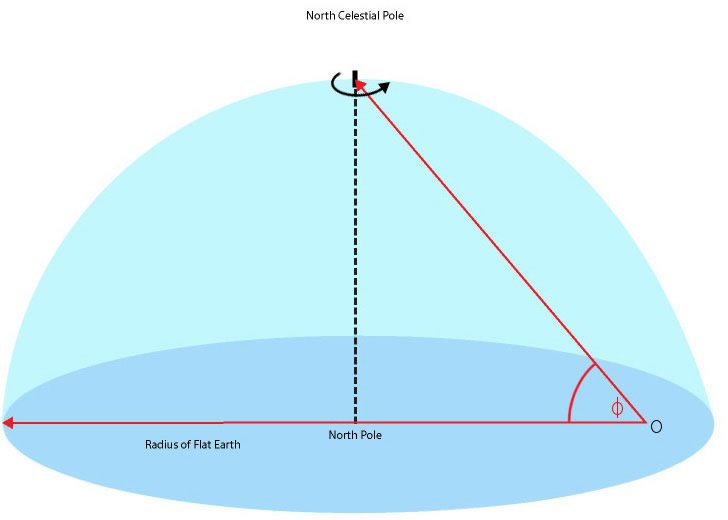
Figure 3.

How well do these predictions of the flat earth model match what we see in the sky? Not very well. The star Polaris is within a degree of the north celestial pole. Being so close to the north celestial pole, Polaris appears to move over a very tiny circle around the north celestial pole. The naked eye generally cannot discern such a small change in position over hours, so Polaris appears to remain motionless over the northern horizon while all other stars appear to spin around it. This is why we call Polaris the North Star. Being so close to the north celestial pole, the North Star can act as a sort of stand-in for the north celestial pole. There is no bright star near the south celestial pole, so there is no South Star. In the Southern Hemisphere, stars appear to spin around the south celestial pole (I have made numerous trips to the Southern Hemisphere and observed this for myself). However, in the flat earth model there is no south celestial pole, so this cannot happen. The simple observation that stars in the Southern Hemisphere indeed appear to spin around the south celestial pole devastatingly disproves the flat earth model.

The North Star is not visible south of the earth’s equator. This is a matter of observational fact. For observers in temperature regions of the Southern Hemisphere, there is a circumpolar region surrounding the north celestial pole that is never visible. This region contains the North Star, the Big Dipper, and the Little Dipper. On a recent rafting trip in the Grand Canyon, a man from Australia was delighted for me to point out the Big Dipper and the North Star, for he had not seen these before. In similar manner, there are stars near the south celestial pole that people in most northern temperate latitudes cannot see. One example is the Southern Cross. Alpha Crucis, the southernmost star in the Southern Cross, is within 27 degrees of the south celestial pole, meaning that it is not visible farther north than 27 degrees north latitude. The northernmost star in the Southern Cross, Gamma Crucis, is 33 degrees from the south celestial pole, so it can be seen no farther north than 33 degrees north latitude. Of course, the stars in question only briefly rise above the horizon at these minimal locations. This fact, along with adverse conditions near the horizon, such as obstructions, would make practical observations very difficult. Even closer to the south celestial pole are two satellite galaxies of the Milky Way, the Large Magellanic Cloud (LMC) and the Small Magellanic Cloud (SMC). While the LMC technically is above the horizon as far north as 18 degrees north latitude and the SMC technically is above the horizon at 16 degrees north latitude, given their diffuse nature, these beautiful objects are not readily visible except in the southern hemisphere.

These circumstances of visibility are easily explained in terms of a spinning spherical earth, as illustrated in Figure 2, because there are circumpolar regions of invisibility in either of earth’s hemispheres. However, with the flat earth model, as in Figure 3, there are no regions of the star dome that are not visible from every location on earth. Therefore, the North Star, the Big Dipper, and the Southern Cross ought to be visible from all locations on the earth. They demonstrably are not visible from all over the earth, so the flat earth model must be false. But there is more.

Star Trails

Long-exposure photographs of stars show that, throughout the night, stars sweep out circular paths centered on the north celestial pole. Proponents of the flat earth frequently claim that these circular star trails cannot be explained with a spinning, spherical earth, but easily can be explained by a flat, non-spinning earth, thus proving the flat earth model (there are many examples of this on the internet; here is just one). Actually, the reverse is true. Here I will demonstrate that the flat earth model cannot explain these motions.

In the accompanying figure, the flat earth is a disk, with the North Pole (indicated by NP) at its center. On the dome of the sky directly above the North Pole is the north celestial pole (NCP). Consider a star at an angular distance of θ from the NCP as observed from the NP. As the dome of the sky rotates each day, the star will sweep out a circle of radius θ as seen from the NP. Consider an observer at point O at some distance from the NP so that the NCP makes an angle φ with the horizon. What shape will the star sweep out from the perspective of an observer at point O? From point O, the circle that the star sweeps out will be projected, so that the observed motion of the star will not be a circle. To see this, consider the angle α, the angle that the star makes with the NCP when closest to point O, and the angle β, the angle that the star makes with the NCP when farthest from point O. Using high school geometry and trigonometry, we find:

By inspection one can see that these angles are not the same, but let us consider a few examples. In each example, let φ be 39 degrees, which is the value of φ where I live in Northern Kentucky. If θ = 10 degrees, α = 3.9 degrees, while β = 2.3 degrees. Notice that this is not a circle, for while the star will appear to orbit the NCP, the star will be farther from the NCP when it appears above the NCP than when it appears below the NCP (a circle, by definition, has constant radius). Next consider a star for which θ = 30 degrees. Now α = 10.7 degrees, while β = 12.5 degrees. Again, this cannot be a circle, because the angles are different. However, notice that unlike before, the star appears closer to the NCP when it appears above the NCP than when it appears below the NCP. If θ = 60 degrees, α = 14.6 degrees, while β = 25.6 degrees. The apparent motion of the star is even more distorted from a circle than in the previous two examples. The point is, since the angles α and β are different, star trails predicted from the flat earth model decidedly are not circular but rather some complicated loop. Since on all the photos of star trails that flat earth proponents post show circles, these photos actually disprove the flat earth model. Their claim is that as the earth rotates there should be a parallax effect. However, we don’t see that because of the incredible distances of stars as compared to the earth’s size.

Equatorial Mounts and Sidereal Drives

Many astronomical telescopes are equipped with equatorial mounts that, when properly aligned with the NCP, allow for a single motion around the polar axis to counteract the earth’s rotation. A sidereal drive is a motor that turns the telescope around the polar axis at a rate of one rotation per sidereal day (about four minutes shorter than a solar day) to keep objects viewed through the telescope centered with no need to adjust position throughout the night. This works very well with the rotating spherical earth with the stars very far away, because in that model stars turn at a uniform rate (one revolution per sidereal day) at the same angular distance from the NCP. However, as demonstrated here, with the flat earth model the angular distance from the NCP would change throughout the night so that stars would drift in the north-south direction. Furthermore, in the flat earth model stars would appear to move in the east-west direction more quickly when observed above the NCP than when observed below the NCP. Therefore, stars would drift in the east-west direction with a sidereal drive if the earth were flat and the stars relatively close to us. I frequently do research with a camera mounted on a telescope with a sidereal drive. This work requires that the telescope precisely track the stars that I observe for many hours. The field of view of the camera typically is 15 arcminutes or less. An arcminute is ¼ of a degree. Obviously, the examples considered here demonstrate that a sidereal drive would do a miserable job of keeping any star in the field of view of the camera, let alone centered, if the flat earth model were true. But this is not the case, so the flat earth model must be false.

The Spherical Earth in a Large Universe

Figure 4 shows how the celestial sphere works in the model where the earth is spherical and the universe is far larger than the earth. An observer’s location is shown on the top of the earth at some latitude φ. The horizon is a horizontal plane tangent to the earth at the observer’s location. The earth’s rotation axis makes an angle φ with horizon. The intersection of the axis with the celestial sphere is the north celestial pole (NCP). If the celestial sphere is very large, the line from the observer’s location to the NCP essentially is parallel to the earth’s rotation axis. Comparison with Figure 2 reveals that Figure 4 is the same. Therefore, the spherical earth, large universe theory consistently explains all phenomena associated with what we observe in the celestial sphere.

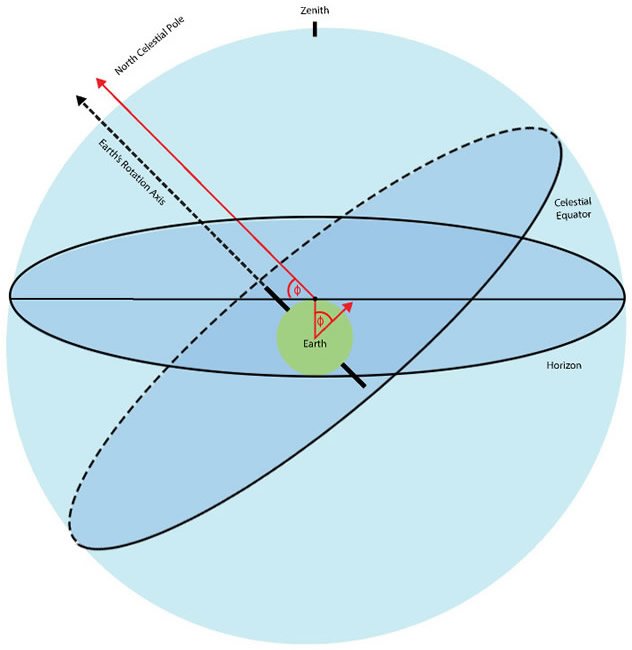
Figure 4.

Conclusion

Here and in my previous discussion I have only scratched the surface of what can be said in response to the flat earth nonsense that is so pervasive today. With time, I will revisit this topic. Skiba chided me for not addressing supposed biblical passages that teach that the earth is flat. If I had dealt with that first, Skiba probably would have criticized me for not dealing with the supposed physical evidence. I am working on a response to what he and others claim the Bible teaches, which I will share eventually. Stay tuned.