
The Cooling of Thick Igneous Bodies on a Young Earth
Abstract
Not only is the presence of water deep in the earth’s crust crucial in producing granitic magmas, but water is also included within such melts. Once a pluton is emplaced (probably rapidly by dikes) and crystallization begun, the magma’s water content significantly aids cooling. Meteoric water also penetrates into the pluton via joints and fractures that develop in the cooled outer rind of the pluton, setting up hydrothermal circulation. The permeability of the cooling pluton is maintained as the cooling/cracking front penetrates inwards, while vapor pressures ensure the fracturing of the surrounding country rocks. Thus, convective cooling rapidly dissipates heat over a timescale compatible with a young earth.
Keywords: granites, plutons, magmas, water contents, conduction, convective cooling, permeability, joints, fractures, hydrothermal activity
This paper was originally published in the Proceedings of the Fourth International Conference on Creationism, pp. 527–545 (1998) and is reproduced here with the permission of the Creation Science Fellowship of Pittsburgh.
Introduction
One of the persistent scientific objections to the earth being young (6,000–7,000 years old rather than 4.55 billion years), and the Flood being a year-long, global event, has been the apparent evidence that large plutons of granitic and other igneous intrusive rocks found today at the earth’s surface necessarily required millions of years to cool from magmas. The purpose of this work is to examine critically this oft-quoted assumption.
Deep in the earth’s lower crust the temperatures are sometimes high enough to melt the rocks locally, particularly if there are applied high pressures due to tectonic forces and/or elevated temperatures. The latter can result from the proximal presence of basaltic magmas ascended from the upper mantle. Most geologists now agree that large “blobs” of granitic magmas are thus generated at 700–900°C, and owing to the fact that these blobs are “lighter” than the surrounding rocks, they are supposed to have risen like balloon-shaped diapirs into the cooler upper crust. There they crystallize into the familiar granitic rocks. When exposed at the earth’s surface due to erosion, these plutons cover large areas, sometimes hundreds of square kilometers. Indeed, it is estimated that up to 86% of the intrusive rocks within the upper continental crust are of granitic composition.1
Young2 has insisted that an immense granitic batholith like that of southern California required a period of about one million years in order to crystallize completely, an estimate repeated by Hayward3 and Strahler4 , the latter in a widely-quoted anti-creationist book. Others quote on the order of 10 million years for the complete process of magma generation, injection, and cooling. Pitcher says:
My guess is that a granitic magma pulse generated in a collisional orogen may, in a complicated way involving changing rheologies of both melt and crust, take 5–10 Ma to generate, arrive, crystallize and cool to the ambient crustal temperature.5
Of course, to this must be added the time to unroof the batholith. However, most recent estimates of these timespans are inflated, as they are based not solely on presumed cooling rates, but primarily on radiometric dating determinations and other uniformitarian assumptions.
Water in Granitic Melts
Recent research in experimental igneous petrology has shown that the temperatures required for melting of rocks to form granitic magmas are significantly lowered by increasing water activity up to saturation, and the amount of temperature lowering increases with increasing pressure.6 A corollary to this is that water solubility in granitic magmas increases with pressure, and therefore depth, so that whereas at 1 kbar pressure (3–4 km depth) the water solubility is 3.7 wt%;7 at 30 kbar pressure (100 km depth) the water solubility is approximately 24 wt%.8 Indeed, the amount of water available is one of three crucial factors in the control of granitic magma formation, the others being parent rock composition and temperature.9 Three sources are believed to provide the needed water—adjacent country rocks, subducted hydrated oceanic crust, and hydrous minerals present in the melting rock itself. These three processes may act either simultaneously or independently of each other. While the adjacent country rocks are of local importance, the other two sources are regarded as supplying large quantities of water.
Water is generally recognized as the most important magmatic volatile component, both for its abundance and for its effects on physical and chemical properties of melts. Indeed, the dramatic effects of the changes of water contents of melts on phase relations is due to the variation of physical properties of melts with changing water content (for example, viscosity, density, diffusivity, solubility of other elements). Therefore, the role of water in melting processes (collecting and segregation of melts), migration of melts, and crystallization of magmas is fundamental.
Experimental investigations have demonstrated that pressure is the most important parameter controlling water solubility in granite petrogenesis, although the influence of temperature and melt composition is also of importance.10 However, water-saturated conditions commonly do not prevail in ascending granitic magmas. Usually they are water-undersaturated, and their viscosity and density thus ensures that they ascend to higher crustal levels where crystallization and cooling of granitic plutons takes place. Thus, if there has been a relatively fast pressure release due to rapid ascent and emplacement of the granitic magma, even if initially water-undersaturated at depth, fast crystallization will occur, and, once water saturation is reached, excess water may be released.
Ascent of Granitic Magmas
There has always been a problem with the accepted “wisdom” of slow magma ascent in balloon-shaped diapirs—the so-called space problem. How does a balloon-shaped diapir with a diameter of several kilometers or more find room to rise through the earth’s crust from 20–40 km (or more) depths and then the space to crystallize there (even at 2–5 km depth) in spite of the continual confining pressures? As Petford, Kerr, and Lister point out,
The established idea that granitoid magmas ascend through the continental crust as diapirs is being increasingly questioned by igneous and structural geologists.11
Clemens and Mawer12 maintain that the idea of long distance diapir transport of granitic magmas is not viable on thermal and mechanical grounds, so they favor the growth of plutons by dike injection propagating along fractures. Pitcher comments:
. . . what is particularly radical is their calculation that a sizeable pluton may be filled in about 900 years. This is really speedy!13
However, Petford, Kerr, and Lister’s calculations show that a crystal-free granitoid melt at 900 °C, with a water content of 1.5 wt%, a viscosity of 8 × 105 Pa s, a density of about 2600 kg/m3 and a density contrast between magma and crust of 200 kg/m3, can be transported vertically through the crust a distance of 30 km along a 6 m wide dike in just 41 days.14 This equates to a mean ascent rate of about 1 cm/sec. At this rate they calculated that the Cordillera Blanca batholith of northwest Peru, with an estimated volume of 6,000 km3, could have been filled from a 10 km long dike in only 350 years. Magma transport has to be at least this fast through such a dike or else the granitic magma would “freeze” due to cooling within the conduit as it ascended. Yet because of the radiometric dating constraints on the fault movements believed responsible for the dike intrusion of the granitic magma, Petford, Kerr, and Lister couldn’t accept this 350 year rapid filling of this batholith, but concluded that the intrusion of the batholith must have been very intermittent, the magma being supplied in brief, catastrophic pulses, while the conduit supposedly remained in place for 3 million years
Epidote is found in some granitic rocks and therefore can have a magmatic origin, its stability in granitic magmas being restricted to pressures of =6 kbar (21 km depth). Brandon, Creaser, and Chacko15 experimental work has shown that epidote dissolves rapidly in granitic melts at pressures of <6 kbar, such that at 700–800°C (temperatures appropriate for granitic magmas) epidote crystals (0.2–0.7 mm) would dissolve within 3–200 years. Therefore, if magma transport from sources in the lower crust were slow (>1,000 years), epidote would not be preserved in upper-crustal batholiths. However, granitic rocks of the Front Range (Colorado) and the White Creek batholith (British Columbia) contain epidote crystals, and Brandon, Creaser, and Chacko found that the 0.5 mm wide epidote crystals in the Front Range granitic rocks would dissolve at 800°C in less than 50 years. They concluded:
Preservation of 0.5 mm crystals therefore requires a transport rate from a pressure of 600 to 200 MPa [6 to 2 kbar] of greater than 700 m year-1.16
This equates to a maximum ascent rate of 14 km per year, which is similar to magma transport rates for dikes based on numerical modeling,17, 18, 19, 20 and close to measured ascent rates for upper crustal magmas.21, 22, 23 By contrast, the modeling of magma transport by ascending diapirs has yielded slow ascent rates of 0.3–50 m per year, meaning ascent times of 10,000–100,000 years.24, 25 In fact, in his widely-quoted anti-creationist book, which is replete with outdated uniformitarian claims, Strahler26 has alleged 150,000 years. In reality, however, the preservation of epidote crystals in some granitic rocks which crystallized at shallow crustal levels not only implies magma transport had to be rapid (very much less than 1,000 years), but that the transport had to be via dikes rather than diapirs.
The mechanical behavior of partially molten granite has been investigated experimentally at temperatures of 800°–1100°C, 250 MPa and confining pressure, different strain rates and under fluid-absent conditions.27 Over that temperature range, strength decreased progressively from 500 MPa to less than 1 MPa. The comparative viscosity of the melt alone was estimated at 950° and 1000 °C from the distance it could be made to penetrate into a porous sand under a known pressure gradient. Rutter and Neumann concluded:
Shear-enhanced compaction is inferred to drive melt into a network of melt-filled veins, whereupon porous flow through the high-permeability vein network allows rapid drainage of melt to higher crustal levels.
Furthermore, it was suggested that the overall kinetics are faster than just gravity-driven porous flow because transport distances to the veins are small and melt pressure gradients, although small, are hundreds to thousands of times higher than those arising in large-scale porous flow. And once the melt accumulates in veins, the effective permeability due to channel flow in the fractures is several orders of magnitude higher than for intergranular flow.
Even more recent experiments have determined the viscosity of Himalayan leucogranite between 800° and 1100°C, 300 and 800 MPa, for meltwater contents of 3.98 and 6.66 wt%.28 The melt viscosity was found to be independent of pressure, and so the experimentally determined phase equilibria constrain the viscosity of this granite to around 104.5 Pa s during its emplacement. It was concluded that
These viscosities and the widths of dikes belonging to the feeder system (20–50 m) are consistent with the theoretical relationship relating these two parameters and show that the precursor magma of the leucogranite was at near liquidus conditions when emplaced within host rocks with preintrusion temperatures around 350°C. Calculated terminal ascent rates for the magma in the dikes are around 1 m/s. Magma chamber assembly time is, on this basis, estimated to be less than 100 years (for a volume of 150 km3). In addition, the dynamical regime of the magma flow in the dikes was essentially laminar, thus allowing preservation of any chemical heterogeneity acquired in the source.
The investigators also noted that repeated injections of magmas over protracted periods will increase the temperatures of the host rocks, and whereas the first injected magmas will traverse cold crust through dikes, later ones will encounter a hotter medium so that the lower viscosity contrast may then be more favorable to diapiric ascent.
Conductive Versus Convective Cooling
Until relatively recently, both intrusives and extrusives were believed to cool primarily by conduction (fig. 1). In the last 20 years or so, however, the role of convective cooling (fig. 1) has become increasingly appreciated.29, 30 In addition, a variety of empirical studies31, 32, 33 have proved that thick igneous bodies do, in fact, cool primarily by circulating water. Cooling models which assume exclusive conductive cooling have been superseded by those which recognize convective cooling in the host rock,34 followed by those which allow for the convective cooling of the outer parts of the plutons also,35 and finally those which allow for constant permeabilities of both host rock and plutons.36, 37, 38
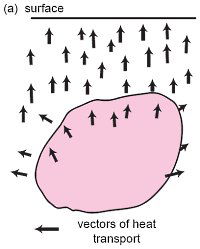
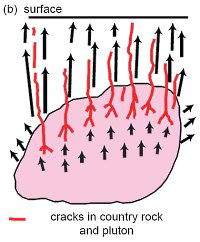
Fig. 1. Cooling of a pluton by (a) conduction or (b) convection. Vectors are proportional to the rate of heat flow to the surface.
The most recent generation of models39 for cooling plutons has been based on the computer program HYDROTHERM.40 Unlike earlier models, this program takes into account the multiphase flow of water, and the heat it carries, at temperatures in the range 0–1200°C and pressures in the range of 0.05–1,000 MPa. Based on a small pluton (2 × 1 km, at 2 km depth), the model indicates that the cooling time is 5,000 years (at a system permeability of 10 md). Increasing the permeability to 33 md shortens the time to only 3,500 years. Unfortunately, however, the program HYDROTHERM has not been applied to plutons of batholitic dimensions. For this reason, our study, described below, must rely on simpler models. Such an approach is justified by the fact that reanalysis of the data used in simpler models, by HYDROTHERM, does not lead to substantially different conclusions regarding inferred cooling time, as predicted by simpler models.41
To begin our study, we must point out that it is at least theoretically possible that some intrusive igneous lithologies had supernatural origins during creation week—some initial rocks had to be supernaturally created with an appearance of a formational history and therefore age. And, of those magmas intruded during the Flood, a large fraction of their latent heat of crystallization may date back to creation and the pre-Flood era. This follows from the fact that 20%–60% of the crystals in a granitic magma may already be crystallized at the time of intrusion.42 Since the latent heat of a crystallizing magma is 65 cal/g and the specific heat is only 0.3 cal/g/°C,43 it follows that an intrusion with 50% pre-crystallization has already, in effect, experienced a built-in cooling of over 100°C.
Furthermore, there is evidence accumulating that many granitic bodies, including the large batholiths, may be essentially “rootless” and not as thick (deep) as the areal extents they cover have seemingly suggested. The subsurface shapes of intrusive igneous masses have been until recently only indirectly known. Mafic bodies appear to have simple dike-like forms extending to great depths, whereas the granitic types are elongate to equant in plan view and extend only to a fraction of their diameters in depth. From a combination of seismic, gravity, and heat flow data, Hamilton and Myers44 suggested that batholithic masses in particular may be only a few kilometers thick. More recently, a seismic reflection study of the internal structure of the English Lake District batholith showed the presence at depth of interpreted granitic sills 500–1,000 m thick separated by country rock, which suggested to Evans et al.45 that the batholith is made up of a series of horizontal sheets with flat tops and floors, or an overall laccolithic structure. Similar sill-like geometries occur in sections of the Sierra Nevada batholith46 and the High Himalaya,47 while the Harney Peak Granite pluton of the Black Hills (South Dakota) has now been mapped as a multiple intrusion that consists of perhaps a few dozen large sills (which are probably no more than 100 m thick, extend laterally for only a few kilometers, and have gentle dips in accord with a domal pattern) and thousands of smaller sills, dikes, and irregularly-shaped intrusions.48 Hutton49 has reviewed granite emplacement mechanisms in three principal tectonic settings and concluded that the plutons have been constructed by multiple granite sheeting parallel to shear zone walls and deformation fabrics. A detailed fractal analysis of the geometries of small to medium laccoliths and plutons50 indicates that they exhibit scale-invariant tabular-sheet geometries, which also implies that larger intrusions (and notably batholiths) are composed of composite sheets of smaller intrusions. This conclusion has been corroborated by a theoretical analysis51 which suggests an upper limit of 2.5 km for the thickness of any single sheet of magma. Finally a variety of geophysical evidences, recently summarized,52 constrain batholiths to total thicknesses of less than 12 km. The implications of all these findings is that many granitic plutons and batholiths consist of relatively thin sills and laccoliths, and hence the time-scale of the apparent crystallization and cooling “problem” is significantly diminished.
The convective overturn caused by settling crystals, in the magma chamber, is a significant factor in the dissipation of its heat. This allows a 10-meter diameter sill to cool in one year53 and, at the other extreme, for a 10 km thick lava “ocean” to theoretically cool in 10,000 years54 by this process alone. In the case of a 2.15 km cuboid pluton which cools by conduction through the country rock (but whose magma can experience convective overturn), its temperature will drop from 850°C to 650°C in 3,000 years55 by this factor alone. If, however, the cooling is exclusively conductive both outside and inside the magma chamber, the time increases to 20,000 years.
Plutons with considerable amounts of magmatic water cool much faster than do those which don’t. Spera has developed a parameterized model for cooling plutons which accounts for heat transfer by conduction and convection within the magma chamber and into the surrounding country rocks.56 According to his model’s central equation linking the crucial parameters, the thermal history of a pluton is most sensitively dependent upon the depth of pluton emplacement, the heat-transfer characteristics of the local environment (for example, emplacement into hydrous or anhydrous country rock), the size of the pluton, and the bulk composition of the melt.
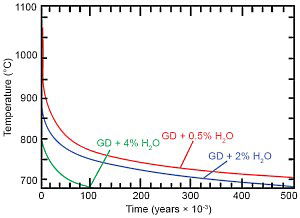
Fig. 2. Influence of magma water content on the cooling history of granodiorite (GD) plutons (radius = 5 km, magma chamber/country rock contact temperature = 600°C, emplacement pressure = 2 kbar). Increasing the water content by a factor of 2 (from 2 to 4 wt%) decreases solidification times by a factor of about 7 (after Spera58).
From the essential results of his study, Spera concluded that emplacement depths and the scale of hydrothermal circulatory systems are first-order parameters in determining the cooling times of large plutons. Fig. 2 shows the “remarkable role” water plays in determining the cooling time. For a granodioritic pluton 10 km wide emplaced at 7 km depth, the cooling time from liquidus to solidus temperatures decreases almost ten-fold as the water content increases from 0.5 wt% to 4 wt%, other factors remaining constant. However, Spera also found that if the temperature of the magma chamber—country rock contact decreases from 700°C to 500°C, which depends on the geothermal gradient, the emplacement depth, and the hydrothermal fluid/magma volume ratio, the cooling time decreases by eighteen-fold (with only 2 wt% water content). Additionally, conduction cooling times were estimated to vary with the square of the radius R, whereas in convective cooling the solidification time varies approximately according to R1.3. Spera concluded:
Hydrothermal fluid circulation within a permeable or fractured country rock accounts for most heat loss when magma is emplaced into water-bearing country rock . . . . Large hydrothermal systems tend to occur in the upper parts of the crust where meteoric water is more plentiful.57
Rock Permeability: The Rate-Determining Factor of Cooling
All of the factors endogenous to the magma itself pale into insignificance, in terms of cooling rate of igneous, once either meteoric or connate water can enter near or into a hot igneous body at an appreciable rate. This is so whether the convection is a “heat engine” driven by the cooling body itself,59 or is a result of extraneous forced convection (discussed below).60 The rate of convective cooling itself scales closely with the rate of water circulated through a temperature anomaly,61 and the volume of water involved in cooling a pluton is less than the volume of the igneous body itself.62
Equation 1 gives the rate of water flux and is a summary of equation (A1) in Cathles.63 The flux rate (Q in Equation 1) is closely proportional to the rate of heat removal from the pluton, since it is the water that carries away virtually all of the heat in a pluton whenever convective cooling dominates over conductive cooling.
Q=[KA (ΔT)/[V] (1)
Q scales with size of intrusion because large intrusions generate proportionately more powerful convective “heat engines.”64 The K refers to permeability of both host rock and igneous body (in millidarcies). The other term, A, encompasses the respective products of other variables (the gravitational constant, the coefficient of thermal expansion of water, and water density changes with depth), whereas V refers to the viscosity of the hydrothermal water. ΔT refers to the elimination of three-quarters of the temperature anomaly between the original temperature of the intrusion and that of the country rock. For instance, if the magma had been intruded at 800°C and the country rock’s temperature had been originally 200°C, there would be 600°C of temperature to eliminate. Thus, in the formula ΔT would equal 450°C. Based on differing geologic conditions, all of the variables in Equation 1, with the exception of permeability K, can change by only about a factor of 2 or so.
The situation is entirely different for permeability K. The permeability K of earth materials varies by several orders of magnitude in crystalline rocks.65 It is thus obvious that the value of Q, and hence the time needed to cool the pluton, is, for all practical purposes, governed by K.
This is borne out by Fig. 3 (which is modified after fig. 6 of Cathles).66 It indicates the time to cool off a pluton of specified transverse dimension as a function of the permeability of the pluton and host rock. (Actually, the cooling in Fig. 3 starts with solidus temperatures and ends at 25% of the difference between ambient temperatures and solidus temperature. The remainder of the cooling to ambient crustal temperatures is not covered by Fig. 3, but is accounted for later.)
The effects of changing permeability K, on cooling time, is striking (fig. 3). An infinitely-long batholith that is 11 km wide, 16.5 km thick, and is buried 20 km below the surface of the ground, when at zero rock permeability (that is, conductive cooling only) needs a few million years to cool. But with the intensity of convective cooling that is allowed by a permeability K of 10 millidarcies (easily exceeded—see below), the time to cool this batholith falls to a mere 3,000 years.
To put this cooling batholith in geothermal perspective, let us consider this: it implies an average geothermal output of 25 W/m2 sustained for the 3,000 years. This pessimistically assumes that the geothermal circulation extends no further than the batholith itself, but thus allows for the presence of parallel batholiths nearby (as is usually the case in orogenic belts), which must undergo their own convective cooling. The quoted heat output is half that of the present-day Grimsvotn geothermal region of Iceland (50 W/m2, sustained over 100 km2 and for 400 years.67
We now perform a sensitivity analysis for the batholithic-emplacement parameters assumed for Fig. 3. Varying the geometry of the pluton (that is, its width-thickness ratio), and its depth of burial relative to its size, from the values arbitrarily chosen for Fig. 3, is relatively unimportant.68 For instance, if the burial depth was doubled, the time to cool would be much less than doubled. Conversely, if it were halved, time to cool would decline by much less than a factor of two. This owes to the fact that the convective cell becomes somewhat more efficient when at greater depth (and vice-versa for shallower depth), and this partly cancels out the increasing (or decreasing) distance which the hot hydrothermal fluid has to travel before reaching the surface and dissipating its heat.
This discussion does not imply that all of the large batholiths had cooled by the time they were uplifted after the Flood. Since most (virtually all?) large batholiths show satellite intrusions and/or pegmatites, this indicates that their centers could have been still liquid at the time they had been uplifted and/or unroofed. And in many areas of the world, geothermal activity from still-hot igneous bodies continues to the present, itself challenging an old earth (see below).
The Extent of Permeability and Hydrothermal Activity
Since the plutons’ cooling rates are essentially limited by rock and crustal permeability, as well as the depth of hydrothermal action, we must go beyond theory and examine how these agents are, in turn, limited under realistic geologic conditions. We also need to understand how these factors came into play during and after the Flood.
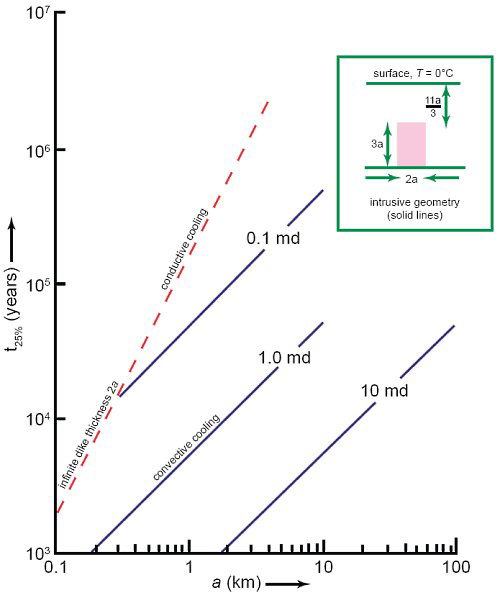
Fig. 3. Diagram showing scaling relationships for identical intrusive geometries cooling by conduction or convection (modified after Cathles74). The particular geometry of the intrusions is indicated in the insert diagram. The solid lines show convective cooling times for different permeabilities (0.1, 1.0 and 10 millidarcies).
By way of introduction, Darcy’s Law allows for the same level of permeability in a rock to be governed by apertures of widely-divergent sizes, and this has been confirmed by actual observations.69 For instance,70 it is clear that a permeability K of 10 md, needed to cool the batholith within 3,000 years (fig. 3), can result from 10-micron microcracks spaced every 0.7 cm, 0.2 mm hairline cracks separated from each other at outcrop scale (100 m), or even 1 mm joints spaced 10 km apart.
Strictly speaking, Darcy’s Law applies to permeable rather than fractured media. However, both theoretical and experimental evidences indicate that fractured domains closely approximate the behavior of equivalent-porous domains when large distances and travel times are involved, when the network of fractures is continuous, and when the heat source which drives the movement of water is large in comparison to the geometry of the fractures and the intervals which separate them.71 These conditions are largely fulfilled by cooling plutons. Moreover, reasonably similar values are obtained for cooling models which allow for a mass flux going through widely-separated fractures of high permeability (and separated by large intervals of low rock-permeability) when contrasted with models which simply compute an equivalent average permeability for the same domain.72, 73 Nevertheless, a residual-cooling model, described below, considers the consequences of the convective cooling, which results from hydrothermal water flowing through widelyseparated (but interconnected) fractures which cut through large thicknesses of otherwise-impermeable rock.
Darcy’s Law is based on the assumption that apertures are perfectly planar and of constant size. Concerns that deviations from these assumptions would cause a severe reduction in actual permeability in the rock have proved unfounded. To begin with, many if not most joints and cracks are reasonably close to planar.75 Moreover, studies on sinuous apertures demonstrate that their permeability is reduced by only about 10–30% over that of perfectly planar ones.76 And while on the subject of theory versus actual geology, it should be noted that convective circulation of the type of interest to us is very difficult to destabilize.77
But how permeable are rocks in actuality? Studies which infer the permeabilities of rocks on a microscopic or hand-sample scale greatly underestimate the permeability of the crust from which they came.78, 79 This indicates that apertures in rocks tend to occur frequently, but at irregular intervals. Thus, the limiting factor now becomes the largest permeability existing over a significant fraction of a given crustal region. This should seldom if ever pose a problem, for joints are virtually universal in granitic terrains,80 as are microfractures.81 In fact, the considerable difficulty of locating granites with low permeability, suitable for long-term storage of radioactive wastes,82 attests to this fact. And, even when located, such bodies often turn out to be dissected by previously-unrecognized joints.83
Among existing granites, the largest K in an area is 1–100 md (not including joints),84 and these values underestimate the permeability it had when hydrothermal solutions circulated through it.85 The latter results from the clogging of apertures during cooling, as is manifested by secondary mineralizations in fractures in the rock.86, 87 There is evidence that presently-impermeable granites were once very permeable, even with microsized apertures. When examined under cathodoluminiscence, seemingly-intact granitic fabrics betray evidence of a former extensive network of microcracks.88
Thus far, we have discussed long-inert granites. By contrast, the permeability of a granite in the actual process of cooling remains unknown. The most recent models assume that an initially-impermeable granite does not become appreciably permeable until it cools below about 360°C, at which time its ductile behavior gives way to brittle behavior, and thus jointing becomes possible.89 Even if this is correct, it need not imply that plutons are virtually impermeable, in a creationist-diluvialist context, when their fabrics are still ductile. Owing to the ubiquity of repeated tectonic stresses as a result of the Flood and its aftermath, combined with the high viscosity of even hot granitic rock, joints will still open up and probably remain open for significant intervals of time before they are “healed” by the flowing of the still-ductile granitic fabric.
These rates of repeated creation of joints (under catastrophic tectonism) and the opposing rates of “healing” in still-ductile rock need to be quantified. An analysis of some of these factors,90 albeit in an actualistic context, suggests the following conclusions: for a sialic pluton at a geothermal gradient of 125°C, and subject to a strain rate of 10-12, the brittle-ductile transition occurs at about 390°C. Under identical conditions, but in the case of a more mafic granitic rock (for example, diorite), the same transition occurs at about 490°C.
Crustal strain rates of 10-13 have been measured after moderate earthquakes, and long-term strain rates on the order of 10-14 are considered plausible.91 It is unclear how much greater the strain rates were during Flood-related tectonism. Assuming that the conditions discussed above, for a sialic pluton, can be validly extrapolated to considerably higher strain rates, the ductile-brittle transition then occurs at approximately 500°C at a strain rate of 10-10, and even, at least theoretically, at approximately 600°C under a strain rate of 10-8.92 However, at such high strain rates, the heating and remelting of crustal material increasingly becomes a factor. More research is needed to understand and quantify these effects.
Both theoretical and experimental evidence indicate that, not only can ostensibly-ductile hot granite behave as a brittle material under sufficient impulsive tectonic stresses, but so can granitic magma itself.93 Moreover, even without the presence of repeated tectonic stresses, and as discussed in the ensuing paragraphs, there are a variety of evidences against a simple ductile/brittle boundary at or about 360°C.
How deep can meteoric water operate? Uniformitarian beliefs had such water limited to only the upper few to several kilometers of the crust, and to crustal temperatures of only a few hundred degrees. Deep boreholes, spaced many thousands of kilometers apart,94, 95 have surprised everyone by revealing that free water exists to at least 12 km depth. They also have contradicted the notion that crustal permeability greatly decreases, if not disappears, at such depths because the overpressure was supposed to crush pores and cracks shut.96 To those who make much of the “testable predictions” claimed for uniformitarian geology, here is yet another example of a set of predictions proved false. Furthermore, seismic data suggest that fractures can exist, at least transiently, down to 15 or 20 km.97
As for temperatures, we now have isotopic evidence that meteoric waters interacted with gabbros, implying temperatures of 500–900°C.98 This also means that such waters can reach the melting zone itself for mafic magmas,99 to say nothing of the cooler sialic magmas which give rise to granites.
Forced Convection and the Removal of Residual Plutonic Heat
We now consider what takes place when the convective cell that cools the pluton has eliminated 75% of the temperature anomaly (fig. 3), and starts to die down. All this time, tectonically and hydrostatically-driven groundwater movement (occurring during and after the Flood) has been taking place, but, until now, has been shunted away by the powerful convection around the pluton. Now, with the “heat engine” petering out,100 the pluton becomes subject to forced convection from the extraneous groundwater migrations.
The limiting factor in the remaining cooling rate now becomes the thinnest distance between parallel watercooling surfaces within the pluton itself. Thus, in order for the remaining heat of the pluton to be dissipated in 2,000 years, the joints allowing free access of water to the pluton need not be spaced any closer than 180 m in a slab-shaped block.101 Under comparable conditions, a 160 m diameter granitic spheroid cools in 2,000 years.102 These computations, however, do not take actual temperature into account. Allowing a joint-dissected pluton to have previously cooled from 850°C (assumed temperature of intrusion) to 650°C, only 2,000 more years are needed to cool conductively the pluton to an ambient crustal temperature of 300°C if each dimension of the cuboid pluton is on the order of 400–500 m.103 This latter computation does not take into account the constant hydrothermal bathing of the cube’s walls.
What if the Apertures Clog Up?
These rough calculations account for the complete closing of microcracks, and thus pessimistically assume zero permeability (and thus exclusive conductive cooling) of the jointed slabs, spheroids, and cubes themselves. Under such restrictions, the convective water cooling is restricted to the jointed surfaces.
However, several factors counteract the sealing of microcracks. One is size. As microcracks approach macroscopic size (1 mm in aperture), they become exponentially more resistant to clogging.104 The common occurrence of partially-filled veins in rock indicates that the sealing of cracks often does not go to completion.105 Also, if the water table fluctuates drastically in an area (as from tectonics), this acts to help keep crustal fractures open.106 Tectonic action counteracts clogging by increasing fluid pressure and causing the flushing out of streamlines, as is manifested by the increase of geothermal activity after even small earthquakes.107 It is also recognized that, where there are high tectonic strain rates, permeabilities at least ten times greater than we have adopted for our model (fig. 3) may be sustained at depth in spite of competing processes such as silica deposition.108 Obviously, such strain rates must have been the norm during and after the Flood, as a consequence of rapid mountain-building, crustal readjustments, etc.
Finally, when cracks do get filled up, they are easily replaced by new ones, especially under catastrophic conditions. Indeed, the rocks which occur in tectonic environments show evidence of repeated generations of mineral-filled fissures and extension cracks, and so permeability of the host rock becomes quickly restored.109 For instance, a new 0.5 cm-wide fracture spaced every 1 km apart will create, or re-create, a crustal permeability K of approximately 10 darcies.110 This is three orders of magnitude greater than that needed for a batholith to cool in 3000 years (see fig. 3). Furthermore, as new joints develop, they allow access of water to hot surfaces. The ensuing cooling generates a new generation of microcracks from the thermoelastic gradients produced by the percolating water.111 Thus, in a sense, both macro- and micro-cracks are self-regenerating, much like the hairs of the fabled Medusa.
Boundary Conditions: Magma and Infiltrating Waters (Extrusives)
Thick lava flows, being surficial, generally cannot develop convection cells as can plutons (see fig. 3). They remain, however, quite vulnerable to cracking and water infiltration. Lister has demonstrated an intense positive feedback process which exists between meteoric water and lava flows (fig. 4).112 Upon contact with water, the lava surface cools rapidly, creating a thin, solid crust. In doing so, a very steep temperature gradient between the recently water-cooled surface (100°C) and the magma just below the surface (1000°C) has been formed, causing severe thermoelastic tension. This soon leads to cracking of the hardened lava crust, allowing water access to the hot interior. So, at once, the lava is cooled to a greater depth, and a new zone of thermoelastic stress is created.

Fig. 4. Feedback between water and a thick lava flow or pluton. Water cooling creates an extreme temperature gradient (shown hatched) in a thin layer of the igneous body. Tensile stresses caused by the gradient lead to further cracking. The cooling front progresses downwards as water enters new cracks and the process repeats itself.
The cycle repeats itself, and the extreme temperature gradient is displaced downwards. All the while, the water-cooled crust is growing thicker, the cracks with their concomitant entry of water keep propagating, and the cooling front is advancing downward. Eventually, the fractures in the lava solidify completely, allowing access to deeply-penetrating water.113 This completes the cooling of the thick lava flow.
Based on calculations, the feedback-generated cooling front can move 5–170 m in a year.114 At the slowest rate, this suffices for cooling the thickest layers of lavas on earth in a few thousand years. Empirical observations on the cooling of a lava lake,115, 116 have demonstrated the movement of a cooling front of over 2 m a year, and further evidence for the importance of this process comes from the heat-production rates of an Icelandic geothermal system.117 It is also recognized that this feedback process explains the occurrence of columnar jointing in basalts,118 and the fact that entablatures in ancient lavas follow downward-growing joints.119
Boundary Conditions: Magma and Circulating Water (Intrusives)
We now focus on processes which make plutons themselves accessible to hydrothermal waters. Consider crustal permeability first. The magma injected into host rock itself exerts pressure upon the host rock, facilitating its fracture,120 and all the more so whenever the intrusion is emplaced rapidly.121 Also, the heat from the pluton122 itself induces fracturing in the country rock as the fluid pressure in the pores of the host rock increase from the heat.123 Upon entry of the pluton’s heat into these new cracks, the process repeats itself.124
Plutons are commonly surrounded by rim monoclines or anticlines. In the past, this has been mistaken as evidence for regional tectonic action. Now it is realized that these regional structures are caused by the fact that, as the plutons cooled, they first weakened the wall rock by giving off heat and fluids.125 Subsequently, the plutons foundered as they cooled, causing the adjacent and superjacent wall rock to buckle downward. Obviously, such a process could only help open up the country rock, and then the pluton itself, to circulating ground water.
Although plutons also rapidly become permeable, let us pessimistically suppose that, unlike the situation discussed in describing Fig. 3, we have permeable crust and a perpetually-impermeable pluton. As before, we have a convective cell, but water cannot enter the pluton itself. So, the heat must leave the pluton itself solely by conduction, and a cooling time of 3000 years (to within 25% of ambient crustal temperatures) suffices for an infinitely-long impermeable pluton which is 0.6 km wide, 0.9 km thick, and 11 km deep.126 For thicker plutons, the limiting factor becomes the spacing of joints. These would have to split the cooling pluton into slabs no larger than 0.6 × 0.9 km, which, as discussed above, is easily met.
Many mineral/metal deposits appear to have been formed by fluids of magmatic-hydrothermal origin associated with granitic and other plutons. Indeed, a granitic magma has enough energy to drive roughly its mass in meteoric fluid circulation.127, 128 Meteoric fluids would thus seem to dominate the magmatic fluid component of even up to 10 wt% or so for some granitic magmas, but several factors can act to focus the magmatic fluids in parts of the hydrothermal system.129 Magmatic fluids are released only while the intrusion is crystallizing, and fractionation of the magmatic fluids to the upper part of the magma chamber can focus their release in a small region of the crust compared with the full extent of the hydrothermal system, so magmatic fluids can locally dominate over meteoric fluids and should not be ignored for the part they can play in cooling plutons.130
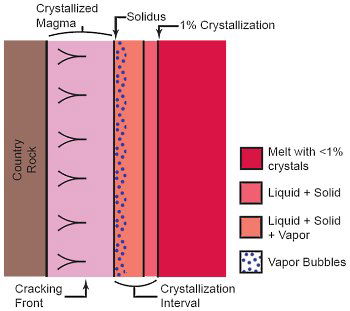
Fig. 5. Cross-section of the margin of a magma chamber traversing (from left to right): country rock, cracked pluton, uncracked pluton, solidus, crystallization interval, and bulk melt (after Candela132).
Following the emplacement of a granitic magma in the upper crust, crystallization occurs due to the irreversible loss of heat to the surrounding country rocks.131 Heat passes out of the magma chamber at the margins of the body, and the solidus moves towards the interior of the chamber, defining an inwardly progressing boundary (fig. 5). As crystallization proceeds, water increases in concentration in the residual melt. When the saturation water concentration is lowered to the actual water concentration in the melt, first boiling occurs and water (as steam) is expelled from solution in the melt, which is driven towards higher crystallinities. Bubbles of water vapor then nucleate and grow, causing second (or resurgent) boiling within the zone of crystallization just underneath the solidus boundary and the already crystallized magma (fi g. 5). As the concentration and size of these vapor bubbles increase, vapor saturation is quickly reached, but initially the vapor bubbles are trapped by the immobile crystallized magma crust.133 The vapor pressure thus increases and the aqueous fluid can then only be removed from the sites of bubble nucleation through the establishment of a three-dimensional critical percolation network, with advection of aqueous fluids through it or by means of fluid flow through a cracking front in the crystallized magma and out into the country rocks. Once such fracturing of the pluton has occurred (and the cracking front will go deeper and deeper into the pluton as the solidus boundary moves progressively inwards towards the core of the magma chamber), not only is magmatic water released from the pluton carrying heat out into the country rocks, but cooler meteoric water in the country rocks is able to penetrate into the pluton and to establish hydrothermal circulation.
There is now petrographic evidence, in the form of complex quartz growth histories,134 which is consistent with the above-discussed sequence of events. Abrupt zone boundaries in quartz crystals indicate fluctuations in melt composition and/or temperature during the crystallization interval.135 In fact, the acceptance of erratic temperature fluctuations in the melt is not favored,136 precisely because of the belief that large plutons “should” cool at slow, continuous rates!
In conclusion, therefore, a long-impermeable pluton is, for our purposes, as unrealistic as it is pessimistic. Pressure build-up within the magma137 will cause the solidified rind of the pluton to crack in short order, resulting in a permeable pluton (fig. 3). The more water dissolved in the magma, the greater will be the pressure exerted at the magma/rock interface.138 If the magma moves in surges, there will be a cyclic cooling and heating of the pluton’s solidified rind, and the resulting thermal stress will exacerbate its cracking.139 As the pluton cools, the cracking front moves progressively deeper into the pluton as the magma/rock boundary recedes inward.
However, this release of magmatic water from a pluton will tend to be focused towards the top or apical region of the magma chamber. The vapor bubbles which nucleate on the magma chamber side-walls will tend to rise as they grow, combining with adjacent vapor bubbles and migrating upwards as a plume towards the top of the magma chamber.140 Because the density of water vapor is reduced as it rises, the buoyancy of the plume is further enhanced, so that this process of bubble-laden plume flow may be a driving force for convection within some magma chambers. Furthermore, the rate of plume flow can be calculated,141 and in the case of a typical granitic magma chamber with 6 wt% water at saturation emplaced at 7–8 km depth, a bubble-laden plume would rise to the top of the pluton in less than a year.142
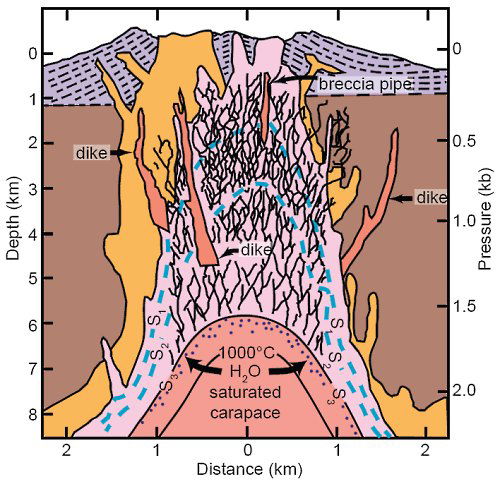
Fig. 6. Schematic cross-section through a hypothetical granodiorite stock at the stage of waning magmatic activity in the development of a porphyry copper (±molybdenum ±gold) system (after Burnham144). A breccia pipe and dikes have formed as a result of wallrock failure, while the chaotic line pattern represents the extensive fracture system developed in the apex above the H2O saturated magma. Note that the granodiorite has intruded into already extruded comagmatic volcanics.
A relevant example that graphically illustrates the rapid release of magmatic fluids through fractures concentrated at the apex of a granitic magma chamber is the typical development of a porphyry copper (±molybdenum ±gold) ore deposit system (fi g. 6), which is now well understood.143 A stock-like body of granodioritic magma is emplaced at shallow depth in a subvolcanic environment, and when water saturation is reached and second boiling occurs the vapor pressure becomes concentrated at the apex or carapace, while the concurrent crystallization process also expands the crystallizing rock mass. The net result is large-scale, brittle fracture of the already crystallized pluton above, so that an intense stockwork of fractures develops into which hydrothermal fluids can flow to deposit their metallic loads (fig. 6). The myriad of fractures is extended upward and outward by continued hydraulic action (hydrofracturing), even into the wallrocks and sometimes the overlying volcanics that were earlier extruded from the same magma chamber—a chimneylike fracture system that channels ore-bearing fluids and heat away from the underlying cooling magma chamber.
Breccia pipes also demonstrate that initiallyimpermeable rinds often fail catastrophically, particularly as a result of the rapid build-up of vapor pressure at the tops of magma chambers.145 We can only underestimate the significance of these intense fracture systems and breccia pipes in the tops of plutons and overlying rocks, as subsequent erosion and the unroofing process must tend to remove them (except in the case of surviving porphyry copper deposits). There is every reason to suppose that this fracturing of the tops of plutons and overlying roof-rocks is ubiquitous, but such fracturing will invariably assist subsequent deep weathering and their rapid erosion and removal to expose the plutons beneath. The same holds for many extrusive equivalents of plutons. The vents responsible for extrusion of comagmatic lavas/volcanics and release of steam and heat from the cooling plutons below have been subsequently eroded away. At other times, however, extrusive equivalents of plutons have only belatedly been recognized to be in genetic association with each other. Such has been the case, for example, for many tuff-batholith associations in the western USA.146
Petrographic evidence147 contradicts the view that plutons remain impermeable for significant periods of time. To the contrary: cracking begins as soon as the quartz is brittle enough, and before the granitic magma has even fully crystallized,148 and continues during its subsequent cooling.149
Finally, the observed rates of geothermal output in modern hydrothermal systems are explicable only if meteoric water has free access to both hot rock and intrusives.150 Note also that the previously-discussed water-induced feedback cooling mechanism of thick lava flows151 must apply to plutons, if only because lithostatic pressure has relatively little effect on the process.152
Rapid Cooling of Igneous Bodies: Geologic Non-Problems
We must now account for the implications of rapid cooling. Consider igneous mineralogy and the belief that relatively large crystals in extrusives mean long cooling times. To begin with, this premise is, on its own terms, inconsistent with the ubiquitous distribution of very tiny crystals in many very thick lava flows,153 which are precisely the ones supposed to take the longest to cool.
Ironic to Young’s argument154 about the slowly-cooling Palisades Sill, it also consists of relatively small crystals and shows evidence of emplacement in 3–4 pulses,155 each of which could have cooled relatively rapidly.156 Of course, the Palisades Sill need not have cooled (or even congealed) within one year (except at its surface) before becoming overlain by fossiliferous rock. In fact, a still-flowing lava soon develops a crust strong enough157 to support a walking person (approximately 770N),158 and so could also support a modest overburden of sediment almost immediately. And, based on analogy with the Kilauea lava,159 a crust of hardened lava a few meters thick can form in a few months, thus being capable of supporting a significant overburden of fossiliferous sediment within that time frame. Consistent with all of these suggestions, the Palisades Sill shows evidence of cooling in both top-down and bottom-up directions,160 as well as evidence for it (and its probable extrusive equivalent) having been deposited subaqueously,161 which, of course, would have greatly accelerated the development of a crust thick enough to support an appreciably-thick superjacent layer of fossiliferous sediments.
It is now recognized that relatively large crystals found in extrusives are not evidence for protracted periods of cooling, if only because these phenocrysts could have formed long before the emplacement of the magma.162 But even in situ crystals can grow rapidly.163 lronically, we now realize that it is the rate of nucleation in the magma, rather than the rate of its cooling, which determines the eventual size of its crystals.164
Contrary to old uniformitarian beliefs, phaneritic textures in granites are not evidence for millions of years of slow cooling. Macroscopic igneous minerals can crystallize and grow to requisite size, in a sialic melt, well within a few thousand years.165, 166 So, for that matter, can phaneritic crystals in a mafic melt.167, 168 It is extraneous geologic factors, not potential rate of mineral growth, which constrain the actual size of crystals attained in igneous bodies.169
Perhaps the most vivid and relevant example is that of granitic pegmatites, regarded as dike-like offshoots of granite plutons because of their spatial associations and identical major mineralogies and bulk compositions.170 At the point of aqueous vapor saturation of a granitic melt, crystal fractionation can sometimes occur, so that volatiles are concentrated in a mobile vapor (hydrothermal)-residual melt phase which readily migrates (usually upwards) into open fractures within the wallrocks immediately adjacent to the granitic pluton, but sometimes within the granite itself.171 It is widely assumed and stated in most textbooks that the giant crystal sizes (sometimes meters long) in pegmatites require very long periods of undisturbed crystal growth, that is, that pegmatite magmas cool slowly. However, London172 noted that constant crystal growth rates of approximately 10-6cm/s could produce quartz and feldspar crystals of pegmatitic dimensions in a few years. Furthermore, in a model of the cooling history of the large Harding pegmatite dike, New Mexico,173 applied conservative boundary conditions (for example, heat loss by conduction only, which is unrealistic) with a magma-wallrock temperature difference of 300°C at emplacement and calculated that the center of the pegmatite dike would have cooled below its equilibrium solidus in about 1–2 years.
What about entablatures and colonnades? It is now recognized that these basaltic textures do not give unambiguous estimates for cooling rates.174 Entablatures form when lavas cool at 1–10°C per hour,175 and colonnades do so at tenfold slower cooling rates. But both estimates are compatible with much higher cooling rates,176 so long as the relative cooling rates of these features differ by at least an order of magnitude.
Convective Cooling of Plutons: Petrographic Signatures
We now examine some of the pitfalls of attempting to minimize the significance of hydrothermal cooling. There is considerable evidence for hydrothermal action (for example, hydrothermal ore deposits, widespread hydrothermal alteration), but absence of evidence for such a process associated with most plutons is not evidence that hydrothermal convective cooling has not occurred. As noted earlier, a major result of hydrothermal action will be intense fracturing in the rocks overlying plutons and the upper zones of plutons themselves, but this has also facilitated erosion and thus removal of the evidence.
Consider secondary hydrous minerals (epidote, chlorite, serpentine, and various clay minerals). Gabbros betray isotopic evidences for hydrothermal alteration, but are “astonishingly free” of such hydrothermal minerals.177 This is because groundwater alteration has occurred at excessive temperatures for these low PT assemblages. Likewise, if certain granites were cooled by hydrothermal fluids at temperatures higher than commonly supposed, there would be no secondary minerals to show this.
However, even this reasoning generously allows for waters cooling the pluton to have experienced free access to its fabric. In actuality, if the fluids flowed mostly through larger cracks or joints, then only the walls of large granitic blocks would show alteration. In fact, such is typical of granites.178 Cathles179 warns against geologists consciously or unconsciously attempting to infer the volume of hydrothermal fluids having circulated through a pluton based on the degree of its alteration. One pluton whose petrographic fabric shows little alteration may have passed a thousand-fold greater volume of hydrothermal fluids than did a second pluton whose fabric shows more alteration than the first!180
Now consider contact metamorphic aureoles. Their size doesn’t give unequivocal evidence for the importance or unimportance of hydrothermal cooling, in spite of models which predict that large aureoles are associated with primarily conductive cooling and small ones result from extensive hydrothermal cooling at high crustal permeabilities.181 The size of the aureole actually shows the maximum distance reached by a certain high temperature emanating from the cooling pluton.182 If, as predicted, microcracks tend to clog as convective cooling proceeds, there may come a time when there is a temporary impermeable cap above the pluton.183 The contact metamorphic aureole would enlarge as the hydrothermal fluids pool and temporarily flow greater distances from the pluton. Furthermore, tectonic effects can perturb, and temporarily expand, circulation streamlines.
Rapid Convective Cooling of Plutons: Isotopic Signatures
An exciting line of evidences for extensive former hydrothermal activity around plutons is provided by the isotopic fractionating of 18O/16O and 2H/1H, and high permeabilities also favor the formation of such signatures.184 Compared with ocean water, meteoric water tends to be isotopically lighter, by several parts per million, and magmatic water tends to be heavier. Therefore, whenever groundwater interacts with plutons, these rocks should be slightly depleted in 18O relative to 16O and, to a less reliable extent, be likewise slightly depleted in deuterium (2H) relative to protium (1H). Large assemblages of plutons, exposed over vast areas (such as the Canadian Cordilleras185) show this.
It is the contrast between isotopic signatures of pluton and host rock that is the most informative. However, the absence of such isotopic signatures need not mean that hydrothermal cooling was unimportant in the history of the pluton, for the following reasons (any of which would have blurred or eliminated the isotopic contrast between pluton and host rock). The magma itself may have been anomalously light isotopically, ground water may have mixed with the magma itself (especially under catastrophic conditions),186 the isotopic signature may have been obscured or erased by subsequent geologic effects,187 etc.
Let us now consider a different hydrological cycle prior to the Flood. Paucity of rainfall facilitates the evaporation of water in landlocked bodies of water, making their isotopes heavier.188 Whenever these waters (and/or their connate water equivalents) had interacted with the cooling plutons, there would be little or no isotopic contrast between them and the magmatic water. Of course, we cannot know the isotopic composition of the connate waters which existed when God had created the earth and which were the first to cool the plutons.
Under such conditions, the first isotopically-light water came into existence only after extensive Flood rainfall had taken place. Upon percolating to great depths and displacing the older, isotopically-heavy connate water, hydrothermal-cooling isotopic signatures were created. Earlier-cooled plutons carry no such signatures despite also having experienced extensive hydrothermal cooling.
Conclusions
With this work, yet another objection to the young-earth creationist position has hopefully been answered. Millions of years are not necessary for the cooling of large igneous bodies. Moreover, the geologic role of hydrothermal cooling has already been extended to account for the rapid origin of thick metamorphic lithologies.189, 190 We now have evidence that regional metamorphism is, thus not unexpectedly, associated with hydrothermal circulation systems which extend 10–100 km from the metamorphic belt itself.191 Moreover, the metamorphic fabric itself can give access to circulating fluids as a result of the microcracking that is now recognized to be a consequence of metamorphic reactions.192 Gabbros themselves can be metamorphosed to the point of acquiring metamorphic hornblende (for example, the so-called amphibolite facies of metamorphism) in a time period as short as a few thousand years down to a few centuries.193
A number of uniformitarian authors194, 195, 196 have pointed out the discrepancy which exists between the large measured permeabilities routinely measured within the earth’s crustal rocks (implying hydrothermal systems having lifetimes of only thousands of years), and the (supposed) need for various geothermal processes to have persisted for millions of years. For this reason, claims have been made about hydrothermal action being episodic and recurring.197, 198, 199 The progressive elimination and rejuvenation of rock permeability, over countless cycles, has also been invoked.200 While, as discussed above, there indeed is much evidence for the previous searing of cracks, the pointed fact is the continued existence of high crustal permeability (primarily cracks and fractures at all scales) in spite of such evidences. Ironically, therefore, hydrothermal cooling not only negates the cooling of plutons as a valid argument for an old earth, but, in and of itself, is more compatible with a young earth.
Future Research
It has been noticed that rapid drops in groundwater levels are sometimes correlated with magmatic activity.201 This needs to be explored in the light of the Flood and its aftermath. The computer model HYDROTHERM,202 thus far applied only to small plutons,203 needs to be employed in order to perform a more sophisticated study of cooling batholiths. Moreover, the composite nature of these large igneous bodies must be better understood and then taken into account in modeling their cooling. The multiphase flow of water simulated by HYDROTHERM also indicates that, as water approaches its critical point (at which time the distinction between liquid water and steam disappears), “superconvection” or “runaway convection” potentially occurs.204 In other words, convective heat transfer becomes suddenly enhanced by a factor of 100 or more. For this to have a chance to take place, a permeability of about 100 md (which is an order of magnitude larger than we have adopted for the cooling batholith: fig. 3) is required,205 along with a narrow window of temperatures. Present evidence suggests that “superconvection” may occur during cooling of small plutons, but probably not of batholiths. Nevertheless, this question must be thoroughly addressed.
More research is needed on the catastrophic extrusion of ancient voluminous lava flows, particularly that which follows up on the following tantalizing lines of evidence: the presence of large vesicles206 in basalts (suggestive of suddenly-trapped volatiles), and textural evidence of very small changes in temperature over considerable distances traveled by extruded lava.207 The latter is true of the Columbia River Basalts (northwest USA), and is consistent with their “extraordinarily rapid emplacement” over an area with a transverse distance of 500 km.208
A major follow-up to this work needs to be a study of economic mineral deposits in the light of the rapid cooling of large igneous bodies. An analogy from the eastern Pacific Ocean provides a fascinating example: massive sulfide deposits of a few tons each have formed, from hydrothermal activity, in less than one year.209
Acknowledgments
Helpful comments and three recent technical references were brought to our attention by Steven A. Austin of the Institute for Creation Research. Other information was provided by several uniformitarian specialists. Additionally, we need to stress that all the work on this paper was our own, including the sourcing of all relevant research papers. However, even though we did not use it, we recognize the “Catastrophe Reference Database” (CATASTROREF) produced by Steven Austin (version 1.2, January 1997) as a very useful source of information on this and many other topics.
Footnotes
- Wedepohl, K. H., 1969. Composition and abundance of common igneous rocks. Handbook of geochemistry (vol. 1, pp. 227–249). Berlin: Springer-Verlag.
- Young, D. A., 1977. Creation and the Flood: An alternative to Flood geology and theistic evolution. Michigan: Baker.
- Hayward, A., 1985. Creation and evolution: The facts and the fallacies. London: Triangle SPCK.
- Strahler, A. N., 1987. Science and earth history—the evolution/creation controversy. New York: Prometheus Books.
- Pitcher, W. S., 1993. The nature and origin of granite. London: Blackie Academic and Professional (p. 187).
- Ebadi, A. and W. Johannes, 1991. Beginning of melting and composition of first melts in the system Qz-Ab-Or-H2O-CO2. Contributions to Mineralogy and Petrology, 106, 286–295.
- Holtz, F., Behrens, H., Dingwell, D. B., and W. Johannes, 1995. Water solubility in haplogranitic melts. Compositional, pressure and temperature dependence. American Mineralogist, 80, 94–108.
- Huang, W. L. and P. J. Wyllie, 1975. Melting reactions in the system NaAlSi3O8-KAISi3O8-SiO2 to 35 kilobars, dry with excess water. Journal of Geology, 83, 737–748.
- Johannes, W. and Holtz, F., 1996. Petrogenesis and experimental petrology of granitic rocks. Berlin: Springer-Verlag (p. 13).
- Johannes and Holtz, Ref. 9, p. 58.
- Petford, N., R. C. Kerr, and J. R. Lister, 1993. Dike transport of granitoid magmas. Geology 21:845.
- Clemens, J. D. and C. K. Mawer, 1992. Granitic magma transport by fracture propagation. Tectonophysics 204:339–360.
- Pitcher, Ref. 5, p. 186.
- Petford, Kerr, and Lister, Ref. 11.
- Brandon, A. D., Creaser, R. A., and T. Chacko, 1996. Constraints on rates of granitic magma transport from epidote dissolution kinetics. Science 271:1845–1848.
- Brandon, Creaser, and Chacko, Ref. 15, p. 1846.
- Clemens and Mawer, Ref. 12.
- Petford, N., 1995. Segregation of tonalitic-trondhjemitic melts in the continental crust: The mantle connection. Journal of Geophysical Research 100:15,735–15,743.
- Petford, N., 1996. Dykes or diapirs? Transactions of the Royal Society of Edinburgh: Earth Sciences 87:105–114.
- Petford, Kerr, and Lister, Ref. 11.
- Chadwick, W. W. Jr., R. J. Archuleta, and A. Swanson, 1988. The mechanics of ground deformation precursory to domebuilding extrusions at Mount St. Helens 1981–1982. Journal of Geophysical Research 93:4351–4366.
- Rutherford, M. J. and P. M. Hill, 1993. Magma ascent rates from amphibole breakdown: An experimental study applied to the 1980–1986 Mount St. Helens eruptions. Journal of Geophysical Research 98:19,667–19,685.
- Scandone, R. and S. D. Malone, 1985. Magma supply, magma discharge and readjustment of the feeding systems of Mount St. Helens during 1980. Journal of Volcanology and Geothermal Research 23:239–262.
- Mahon, K. I., T. M. Harrison, and D. A. Drew, 1988. Ascent of a granitoid diapir in a temperature varying medium. Journal of Geophysical Research 93:1174–1188.
- Weinberg, R. F. and Y. Podladchikov, 1994. Diapiric ascent of magmas through Power Law crust and mantle. Journal of Geophysical Research 99:9543–9559.
- Strahler, Ref. 4.
- Rutter, E. H. and D. H. K. Neumann, 1995. Experimental deformation of partially molten Westerly Granite under fluid-absent conditions, with implications for the extraction of granitic magmas. Journal of Geophysical Research 100: 15,697–15,715.
- Scaillet, B., F. Holtz, M. Pichavant, and M. Schmidt, 1996. Viscosity of Himalayan leucogranites: Implications for mechanisms of granitic magma ascent. Journal of Geophysical Research 101:27,691–27,699.
- Norton, D., 1978. Sourcelines, sourceregions, and pathlines for fluids in hydrothermal systems related to cooling plutons. Economic Geology 73:21–28.
- Taylor, H. P., 1990. Oxygen and H-isotope constraints on the deep circulation of surface waters into zones of hydrothermal metamorphism and melting. The role of fluids in crustal processes, National Research Council (USA) (pp. 72–95). Washington, DC: National Academy Press.
- Brown, S. R., 1987. Fluid flow through rock joints: The effect of surface roughness. Journal of Geophysical Research 92: 1337–1347.
- Hardee, H. C., 1980. Solidification in Kilauea lki Lava Lake. Journal of Volcanology and Geothermal Research 7:211–223.
- Hardee, H. C., 1982. Permeable convection above magma bodies. Tectonophysics 84:179–195.
- Cheng, P. and W. J. Minkowycz, 1977. Free convection about a vertical flat plate embedded in a porous medium with application to heat transfer from a dike. Journal of Geophysical Research 82(14):2040–2044.
- Norton, D. and J. Knight, 1977. Transport phenomena in hydrothermal systems: Cooling plutons. American Journal of Science 277:937–981.
- Cathles, L. M., 1981. Fluid flow and genesis of hydrothermal ore deposits. In , Skinner, B. J. (ed.), Economic geology: 75th anniversary volume, pp. 424–457.
- Parmentier, E. M., 1981. Numerical experiments on 18O depletion in igneous intrusions cooling by groundwater convection. Journal of Geophysical Research 86:7131–7144.
- Torrance, K. E. and J. P. Sheu, 1978. Heat transfer from plutons undergoing hydrothermal cooling and thermal cracking. Numerical Heat Transfer 1:147–161.
- Hayba, D. O. and S. E. lngebritsen, 1997. Multiphase groundwater flow near cooling plutons. Journal of Geophysical Research 102:12,235–12,252.
- lngebritsen, S. E. and D. O. Hayba, 1994. Fluid flow and heat transport near the critical point of H2O. Geophysical Research Letters 21:2199–2202.
- Hayba and Ingebritsen, Ref. 39.
- Taylor, Ref. 30.
- White, D. F. and D. I. Williams, 1975. Assessment of geothermal resources of the United States—1975. United States geological survey circular 726.
- Hamilton, W. and W. Myers, 1967. The nature of batholiths. United States Geological Survey Professional Paper 554C.
- Evans, D. J., W. J. Rowley, R. A. Chadwick, G. S. Kimbell, and D. Millward, 1994. Seismic reflection data and the internal structure of the Lake District Batholith, Cumbria, Northern England. Proceedings of the Yorkshire Geological Society 50: 11–24.
- Coleman, D. S., A. F. Glazner, J. S. Miller, K. J. Bradford, T. P. Frost, J. L. Joye, and C. A. Bachl, 1995. Exposure of a Late Cretaceous layered mafic-felsic magma system in the Central Sierra Nevada Batholith, California. Contributions to Mineralogy and Petrology 120:129–136.
- Scaillet, B., A. Pêcher, P. Rochette, and M. Champenois, 1995. The Gangotri Granite (Garhwal Himalaya): Laccolithic emplacement in an extending collisional belt. Journal of Geophysical Research 100:585–607.
- Norton, J. J. and J. A. Redden, 1990. Relations of zoned pegmatites to other pegmatites, granite, and metamorphic rocks in the Southern Black Hills, South Dakota. American Mineralogist 75:631–655.
- Hutton, D. H. W., 1992. Granite sheeted complexes: Evidence for the dyking ascent mechanism. Transactions of the Royal Society of Edinburgh: Earth Sciences 83:377–382.
- McCaffrey, K. J. W. and N. Petford, 1997. Are granitic intrusions scale invariant? Journal of the Geological Society of London 154:1–4.
- McCaffrey and Petford, Ref. 50.
- McCaffrey and Petford, Ref. 50.
- Carrigan, C. R., 1983. A heat pipe model for vertical, magma-filled conduits. Journal of Volcanology and Geothermal Research 16:279–298.
- Marsh, B. D., 1989. Convective style and vigour in magma chambers. Journal of Petrology 30(3):479–530.
- Smith, R. L. and H. R. Shaw, 1979. Igneous-related geothermal systems. United States Geological Survey Circular 790.
- Spera, F. J., 1982. Thermal evolution of plutons: A parameterized approach. Science 207:299–301.
- Spera, Ref. 56, p. 299.
- Spera, Ref. 56.
- Cathles, Ref. 36.
- Bodvarsson, G. and R. P. Lowell, 1972. Ocean-floor heat flow and the circulation of interstitial waters. Journal of Geophysical Research 77:4472–4475.
- Cathles, Ref. 36.
- Cathles, Ref. 36.
- Cathles, Ref. 36.
- Cathles, L. M., 1977. An analysis of the cooling of intrusives by ground-water convection which includes boiling. Economic Geology 72:804–826.
- Brace, W. F., 1980. Permeability of crystalline and argillaceous rocks. Journal of Rock Mechanics 17:241–251.
- Cathles, Ref. 36.
- Bjornnsson, H., S. Bjornnsson, and T. Sigurgeirsson, 1982. Penetration of water into hot rock boundaries of magma at Grimsvotn. Nature 295:580–581.
- Cathles, Ref. 36.
- Fehn, Y., L. M. Cathles, and H. D. Holland, 1978. Hydrothermal convection and uranium deposits in abnormally radioactive plutons. Economic Geology 73:1556–1566.
- Norton, D. and R. Knapp, 1977. Transport phenomena in hydrothermal systems: The nature of porosity. American Journal of Science 277:913–936.
- Wanfang, Z., H. S. Wheater, and P. M. Johnson, 1997. State of the art of modelling two-phase flow in fractured rock. Environmental Geology 31:157–166.
- Fehn, U. and L. M. Cathles, 1979. Hydrothermal convection at slow-spreading mid-ocean ridges. Tectonophysics 55:239–260.
- Fehn, Cathles, and Holland, Ref. 69.
- Cathles, Ref. 36.
- Lister, C. R. B., 1976. On the penetration of water into hot rock. Journal of the Royal Astronomical Society 39:465–509.
- Brown, Ref. 31.
- Murphy, H. D., 1979. Convective instabilities in vertical fractures and faults. Journal of Geophysical Research 84: 6121–6130.
- Brace, Ref. 65.
- Norton and Knapp, Ref. 70.
- Secor, D. T., 1965. Role of fluid pressure in jointing. American Journal of Science 263:633–646.
- Bauer, S. J. and J. Handin, 1983. Thermal expansion and cracking of three confined, water-saturated igneous rocks to 800 °C. Rock mechanics and rock engineering, pp. 181–198.
- Green, A. G. and J. A. Mair, 1983. Subhorizontal fractures in a granitic pluton: Their detection and implications for radioactive waste disposal. Geophysics 48:1428–1449.
- Green and Mair, Ref. 82.
- Brace, Ref. 65.
- Long, P. E. and B. J. Wood, 1986. Structures, textures, and cooling histories of Columbia River Basalt flows. Geological Society of America Bulletin 97:1144–1155.
- Ramsay, J. G., 1980. The crack-seal mechanism of rock deformation. Nature 284:135–139.
- Long and Wood, Ref. 85.
- Sprunt, E. S. and A. Nur, 1979. Microcracking and healing in granites: New evidence from cathodoluminescence. Science 205:495–497.
- Ingebritsen and Hayba, Ref. 40.
- Fournier, R. O., 1991. The transition from hydrostatic to greater than hydrostatic fluid pressure in presently active continental hydrothermal systems in crystalline rock. Geophysical Research Letters 18:955–958.
- Fournier, Ref. 90.
- Fournier, Ref. 90, Fig. 1, left.
- Dingwell, D. B., 1997. The brittle-ductile transition in high-level granitic magmas: Material constraints. Journal of Petrology 38:1635–1644.
- Kerr, R. A., 1993. Looking deeply into the earth’s crust in Europe. Science 261:295–297.
- Kerr, R. A., 1994. German super-deep hole hits bottom. Science 266:545.
- Kerr, Ref. 94.
- Norton and Knapp, Ref. 70.
- Scaillet et al., Ref. 28.
- Scaillet et al., Ref. 28.
- Cathles, Ref. 64.
- Bodvarsson and Lowell, Ref. 60.
- Norton, Ref. 29.
- Smith and Shaw, Ref. 55.
- Smith, D. L. and B. Evans, 1984. Diffusional crack healing in quartz. Journal of Geophysical Research 89(B6):4125–4135.
- Knapp, R. B. and D. Norton, 1981. Preliminary numerical analysis of processes related to magma crystallization and stress evolution in cooling pluton environments. American Journal of Science 281:35–68.
- Bjornnsson, Bjornnsson, and Sigurgeirsson, Ref. 67.
- White, D. E., 1968. Hydrology, activity, heat flow of the Steamboat Springs Thermal System. United States Geological Survey Professional Paper 458-C.
- Ingebritsen and Hayba, Ref. 40.
- Ramsay, Ref. 86.
- Spooner, E. T. C., 1977. Hydrodynamic model for the origin of the ophiolitic cupriferous pyrite ore deposits of Cyprus. Geological society of London special publication no. 7, pp. 58–71.
- Zhao, J. and E. T. Brown, 1992. Thermal cracking induced by water flow through joints in heated granite. International Journal of Rock Mechanics 17:77–82.
- Lister, Ref. 74.
- Reiter, M., M. W. Barroll, J. Minier, and G. Clarkson, 1987. Thermo-mechanical model for incremental fracturing in cooling lava flows. Tectonophysics 142:241–260.
- Lister, Ref. 75.
- Hardee, Ref. 32.
- Hardee, Ref. 33.
- Bjornnsson, Bjornnsson, and Sigurgeirsson, Ref. 67.
- Reiter et al., Ref. 113.
- DeGraff, J. M., P. E. Long, and A. Aydin, 1989. Use of joint-growth directions and rock textures to infer thermal regimes during solidification of basaltic lava flows. Journal of Volcanology and Geothermal Research 38:309–324.
- Knapp and Norton, Ref. 105.
- Delaney, P. T. 1982. Rapid intrusion of magma into wet rock: Groundwater flow due to pore pressure increases. Journal of Geophysical Research 87:7739–7756.
- Fehn, Cathles, and Holland, Ref. 69.
- Knapp, R. B. and J. E. Knight, 1977. Differential thermal expansion of pore fluids: Fracture propagation and microearthquake production in hot plutonic environments. Journal of Geophysical Research 82:2515–2522.
- Knapp and Knight, Ref. 123.
- Glazner, A. F. and D. M. Miller, 1996. Late-stage sinking of plutons. Geology 25:1099–1102.
- Cathles, Ref. 36.
- Norton, D. L. and L. M. Cathles, 1979. Thermal aspects of ore deposition. In Barnes, H. L. (ed.), Geochemistry of hydrothermal ore deposits, 2nd ed., pp. 611–631. New York: Wiley.
- Cathles, Ref. 36.
- Burnham, C. W., 1997. Magmas and hydrothermal fluids. In Barnes, H. L. (ed.), Geochemistry of hydrothermal ore deposits, 3rd ed., pp. 63–123. New York: Wiley.
- Hanson, R. B. (1996). Hydrodynamics of magmatic and meteoric fluids in the vicinity of granitic intrusions. Transactions of the Royal Society of Edinburgh: Earth Sciences 87:251–259.
- Candela, P. A., 1992. Controls on ore metal ratios in granite-related ore systems: An experimental and computational approach. Transactions of the Royal Society of Edinburgh: Earth Sciences 83:317–326.
- Candela, P. A., 1991. Physics of aqueous phase evolution in plutonic environments. American Mineralogist 76:1081–1091.
- Candela, Ref. 132.
- D’Lemos, R. S. et al., 1997. Complex quartz growth histories in granite revealed by scanning cathodoluminescence techniques. Geological Magazine 134:549–552.
- D’Lemos, Ref. 134.
- D’Lemos, Ref. 134.
- Delaney, Ref. 121.
- Knapp and Norton, Ref. 105.
- Bauer and Handin, Ref. 81.
- Candela, Ref. 132.
- Marsh, B. D., 1988. Crystal capture, sorting, and retention in convecting magma. Geological Society of America Bulletin 100: 1720–1737.
- Candela, Ref. 132.
- Burnham, Ref. 128.
- Burnham, Ref. 128.
- Norton, D. L. and L. M. Cathles, 1973. Breccia pipes—products of exsolved vapor from magmas. Economic Geology 68:540–546.
- Lipman, P. W., 1984. The roots of ash flow calderas in western North America: Windows into the tops of granitic batholiths. Journal of Geophysical Research 89:8801–8841.
- Sprunt and Nor, Ref. 88.
- Sprunt and Nor, Ref. 88.
- Sprunt and Nor, Ref. 88.
- Bjornnsson, Bjornnsson, and Sigurgeirsson, Ref. 67.
- Lister, Ref. 75.
- Lister, Ref. 75.
- Marsh, Ref. 54.
- Young, Ref. 2.
- Shirley, D. S., 1987. Differentiation and compaction in the Palisades Sill, New Jersey. Journal of Petrology 28:835–865.
- Hardee, Ref. 32.
- Williams, H. and A. R. McBirney, 1979. Volcanology. San Francisco: Freeman, Cooper and Co.
- Campbell, C. I., 1996. Dynamic analysis of the Maribrynong River footbridge. Proceedings of the 1996 wood engineering conference, pp. 225–231.
- Hardee, Ref. 32.
- Shirley, Ref. 155.
- Shirley, Ref. 155.
- Marsh, Ref. 54.
- Tyler, D. J., 1990. Tectonically-controlled rock cycle. In Walsh, R. E. and C. L. Brooks (eds.), Proceedings of the second international conference on creationism, vol. 2, pp. 293–299. Pittsburgh, Pennsylvania: Creation Science Fellowship.
- Tsuchiyama, A., 1983. Crystallization kinetics in the system CaMgSi2O6-CaAl2Si2O8: The delay in nucleation of diopside and anorthite. American Mineralogist 68:687–698.
- Swanson, S. E., 1977. Relation of nucleation and crystal-growth rate to the development of granitic textures. American Mineralogist 62:996–978.
- Swanson, S. E. and P. M. Fenn, 1986. Quartz crystallization in igneous rocks. American Mineralogist 71:331–342.
- Brandeis, G. and C. Jaupart, 1987. The kinetics of nucleation and crystal growth and scaling laws for magmatic crystallization. Contributions to Mineralogy and Petrology 96:24–34.
- 168. Dunbar, N. W., G. K. Jacobs, and M. T. Naney, 1995. Crystallization processes in an artificial magma: Variations in crystal shape, growth rate, and composition with melt cooling history. Contributions to Mineralogy and Petrology 120:412–425.
- Marsh, Ref. 54.
- London, D., 1996. Granitic pegmatites. Transactions of the Royal Society of Edinburgh: Earth Sciences 87:305–319.
- Jahns, R. H. and C. W. Burnham, 1969. Experimental studies of pegmatite genesis: 1. A model for the derivation and crystallization of granitic pegmatites. Economic Geology 64:843–864.
- London, D., 1992. The application of experimental petrology to the genesis and crystallization of granitic pegmatites. Canadian Mineralogist 30:499–540.
- Chakoumakos, B. C. and G. R. Lumpkin, 1990. Pressure-temperature constraints on the crystallization of the Harding Pegmatite, Taos County, New Mexico. Canadian Mineralogist 28:287–298.
- DeGraff, Long, and Aydin, Ref. 119.
- Long and Wood, Ref. 85.
- Taylor, Ref. 30.
- Norton, D. and H. P. Taylor, 1979. Quantitative simulation of the hydrothermal systems of crystallizing magmas on the basis of transport theory and oxygen isotope data. Journal of Petrology 20:421–486.
- Cathles, L. M., 1991. The importance of vein selvaging in controlling the intensity and character of subsurface alteration in hydrothermal systems. Economic Geology 86:466–471.
- Norton and Knapp, Ref. 70.
- Parmentier, E. M. and A. Schedl, 1981. Thermal aureoles of igneous intrusions: Some possible indications of hydrothermal convective cooling. Journal of Geology 89:1–22.
- Parmentier and Schedl, Ref. 180.
- Cathles, Ref. 36.
- Parmentier and Schedl, Ref. 180.
- Magaritz, M. and H. P. Taylor, H. P., 1986. Oxygen 18/oxygen 16 and D/H studies of plutonic granitic and metamorphic rocks across the Cordilleran Batholiths of Southern British Columbia. Journal of Geophysical Research 91, 2193–2217.
- Hildreth, W., R. L. Christiansen, and J. R. O’Neil, 1984. Catastrophic isotopic modification of rhyolitic magma at times of caldera subsidence, Yellowstone Plateau volcanic field. Journal of Geophysical Research 89:8339–8369.
- Taylor, Ref. 30.
- Taylor, H. P., 1974. The application of oxygen and hydrogen isotope studies to problems of hydrothermal alteration and ore deposition. Economic Geology 69:843–883.
- Snelling, A. A., 1994a. Towards a creationist explanation of regional metamorphism. Creation Ex Nihilo Technical Journal 8:51–77.
- Snelling, A. A., 1994b. Regional metamorphism within a creationist framework: What garnet compositions reveal. In Walsh, R. E., (ed.), Proceedings of the third international conference on creationism, pp. 485–496. Pittsburgh, Pennsylvania: Creation Science Fellowship.
- Garven, G., 1995. Groundwater flow and continental-scale geologic processes. Annual Review of Earth and Planetary Sciences 23:89–117.
- Connolly, J. A. D. et al., 1997. Reaction-induced microcracking: An experimental investigation of a mechanism for enhancing anatectic melt extraction. Geology 25:591–594.
- Manning, C. E., P. E. Weston, and K. I. Mahon, 1996. Rapid high-temperature metamorphism of East Pacific Rise gabbros from Hess Deep. Earth and Planetary Science Letters 144:123–132.
- Cathles, Ref. 36.
- Elder, J. W., 1977. Model of hydrothermal ore genesis. Geological society of London special publication no. 7, pp. 4–13.
- Silberman, M. L., D. E. White, T. E. C. Keith, and R. D. Dockter, 1979. Duration of hydrothermal activity at Steamboat Springs, Nevada, from ages of spatially associated volcanic rocks. United States Geological Survey Professional Paper 458-D.
- Cathles, L. M., 1990. Scales and effects of fluid flow in the upper crust. Science 248:323–329.
- Nur, A. and J. Walker, 1990. Time-dependent hydraulics of the earth’s crust. The role of fluids in crustal processes, National Research Council (USA), pp. 113–127. Washington, DC: National Academy Press.
- Silberman et al., Ref. 195.
- Cathles, Ref. 36.
- Bjornnsson, Bjornnsson, and Sigurgeirsson, Ref. 67.
- Ingebritsen and Hayba, Ref. 41.
- Hayba and Ingebritsen, Ref. 40.
- Ingebritsen and Hayba, Ref. 41.
- Ingebritsen and Hayba, Ref. 41.
- Ingebritsen and Hayba, Ref. 4.
- Gray, N. H. and R. A. Simmons, 1985. Half-moon vesicles in the Mesozoic Basalts of the Hartford Basin: Their origin and potential use as paleoflow indicators. Geological Society of America Abstracts with Programs 17:21.
- Ho, A. M. and K. V. Cashman, 1997. Temperature constraints on the Ginkgo Flow of the Columbia River Basalt Group. Geology 25:403–406.
- Ho and Cashman, Ref. 207.
- Shanks, W. C. et al., 1995. Re-birth of hydrothermal vent systems and growth of sulfide deposits following the 1991 Ridge Crest Eruption, 9–10 °N East Pacific Rise. Geological Society of America Abstracts with Programs 27:A-280.
Recommended Resources

Answers in Genesis is an apologetics ministry, dedicated to helping Christians defend their faith and proclaim the good news of Jesus Christ.
- Customer Service 800.778.3390
- © 2025 Answers in Genesis




